ARTICLE 58

by
Stephen M. Phillips
Flat 4,
Oakwood House, 117-119 West Hill Road. Bournemouth. Dorset BH2 5PH.
England.
Website: http://smphillips.mysite.com
Abstract
|
The inner form of the Tree of Life consists of two
sets of seven regular polygons, one set being the mirror image of the
other. The polygon in either set that has the most corners is the
dodecagon. As it is the tenth type of polygon, three types of polygons
are absent from the inner Tree of Life. Previous articles have shown
that its seven types of polygons encode information about how this
blueprint manifests in holistic systems. If they formally correspond to
the seven Sephiroth of Construction, this suggests that the three absent
polygons may correspond to the three members of the Supernal Triad, so
that the first 10 regular polygons correspond to the 10 Sephiroth. This
article uncovers amazing evidence of design, i.e., conceptual coherence,
rather than unconnected disorder, that supports this conjecture. Data is
provided in the form of the geometrical and yod compositions of the
first ten regular polygons. They are found to embody the fine-structure
number 137, the superstring structural parameters 168 & 840 and the
dimension 496 of the two superstring gauge symmetry groups SO(32) &
E8×E8.
|
1
Table 1. Gematria number values of the ten Sephiroth in the four
Worlds.
| |
SEPHIRAH
|
GODNAME
|
ARCHANGEL
|
ORDER OF
ANGELS
|
MUNDANE
CHAKRA
|
|
1
|
Kether
(Crown)
620 |
EHYEH
(I am)
21 |
Metatron
(Angel of the
Presence)
314 |
Chaioth ha Qadesh
(Holy Living
Creatures)
833
|
Rashith ha Gilgalim
First Swirlings.
(Primum Mobile)
636 |
|
2
|
Chokmah
(Wisdom)
73 |
YAHWEH, YAH
(The Lord)
26,
15
|
Raziel
(Herald of the
Deity)
248 |
Auphanim
(Wheels)
187 |
Masloth
(The Sphere of
the Zodiac)
140 |
|
3
|
Binah
(Understanding)
67 |
ELOHIM
(God in multiplicity)
50
|
Tzaphkiel
(Contemplation
of God)
311
|
Aralim
(Thrones)
282
|
Shabathai
Rest.
(Saturn)
317 |
| |
Daath
(Knowledge)
474 |
|
|
|
|
|
4
|
Chesed
(Mercy)
72 |
EL
(God)
31 |
Tzadkiel
(Benevolence
of God)
62 |
Chasmalim
(Shining Ones)
428
|
Tzadekh
Righteousness.
(Jupiter)
194 |
|
5
|
Geburah
(Severity)
216
|
ELOHA
(The Almighty)
36
|
Samael
(Severity of God)
131
|
Seraphim
(Fiery Serpents)
630
|
Madim
Vehement Strength.
(Mars)
95 |
|
6
|
Tiphareth
(Beauty)
1081
|
YAHWEH ELOHIM
(God the Creator)
76 |
Michael
(Like unto God)
101
|
Malachim
(Kings)
140
|
Shemesh
The Solar Light.
(Sun)
640 |
|
7
|
Netzach
(Victory)
148
|
YAHWEH SABAOTH
(Lord of Hosts)
129
|
Haniel
(Grace of God)
97 |
Tarshishim or
Elohim
1260
|
Nogah
Glittering Splendour.
(Venus)
64 |
|
8
|
Hod
(Glory)
15
|
ELOHIM SABAOTH
(God of Hosts)
153
|
Raphael
(Divine Physician)
311
|
Beni Elohim
(Sons of God)
112
|
Kokab
The Stellar Light.
(Mercury)
48 |
|
9
|
Yesod
(Foundation)
80
|
SHADDAI EL CHAI
(Almighty Living
God)
49,
363
|
Gabriel
(Strong Man of
God)
246
|
Cherubim
(The Strong)
272
|
Levanah
The Lunar Flame.
(Moon)
87 |
|
10
|
Malkuth
(Kingdom)
496
|
ADONAI MELEKH
(The Lord and King)
65,
155
|
Sandalphon
(Manifest Messiah)
280 |
Ashim
(Souls of Fire)
351
|
Cholem Yesodoth
The Breaker of the
Foundations.
The Elements.
(Earth)
168 |
|
The Sephiroth exist in the four Worlds of Atziluth, Beriah,
Yetzirah and Assiyah. Corresponding to them are the Godnames,
Archangels, Order of Angels and Mundane Chakras (their physical
manifestation). This table gives their number values obtained by the
ancient practice of gematria, wherein a number is assigned to each
letter of the alphabet, thereby giving a number value to a word that is
the sum of the numbers of its letters.
|
(Numbers in this table referred to in the article will be written in
boldface).
2
1.
The 2nd-order Tetractys Pattern in the
First 10 Polygons
The inner form of the Tree of Life
comprises two similar sets of seven enfolded, regular polygons, one the mirror image of
the other:
triangle, square, pentagon,
hexagon, octagon, decagon & dodecagon.
The dodecagon is the tenth regular polygon, so that three polygons are absent
from the inner Tree of Life: the 7-sided heptagon, the 9-sided nonagon & the
11-sided undecagon. They are shown coloured white in Figure 1, the seven other types of
polygons belonging to the inner Tree of Life being coloured the seven colours of the
rainbow. This absence suggests the following analogy between the first 10 regular
polygons and the 10 Sephiroth of the Tree of Life:
-
the three types of "absent
polygons" → three Sephiroth belonging to the Supernal Triad, which express the
subjective, 3-fold aspect of God (the triple Godhead) and which stand outside
all levels of reality;
-
the seven types of
polygons making up the inner Tree of Life → seven Sephiroth of
Construction, which express the objective, 7-fold aspect of God and which
manifest in His Creation.
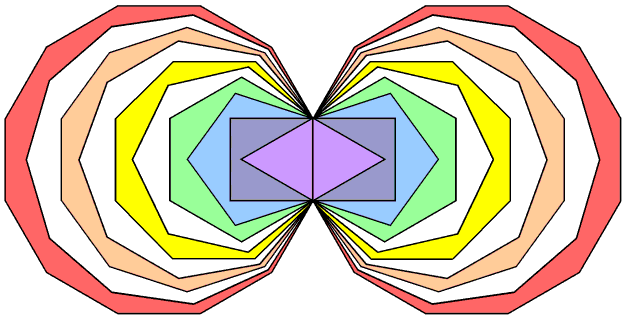 Figure 1.
The first (10+10) enfolded polygons. Figure 1.
The first (10+10) enfolded polygons.
Despite the fact that the geometry of
overlapping circles that generates the inner Tree of Life
(see here)
does not create three of the first 10 regular polygons, these absent polygons, being
analogous to the archetypal Supernal Triad as the source of all levels of existence,
might be expected to have properties that are analogous to those found in the seven
types of polygons, if this analogy between Sephiroth and polygons really holds. Let us
now investigate whether this is true.
The number of corners of the
n sectors of an n-gon = n + 1. The separate heptagon (n=7), nonagon (n=9) &
undecagon (n=11) have 27 sectors with 30 corners. Enfolded, they have
(30−2−2=26)
corners, the two mirror-image sets having 50 corners, where
26 is the
number value of YAHWEH, the Godname of Chokmah, and 50 is the number value of ELOHIM,
the Godname of Binah. These two Sephiroth head the Pillars, respectively, of Mercy and
Judgement in the Tree of Life. As such, their Godnames prescribe the
 Figure 2. The 2nd-order tetractys character of the first 10
separate polygons.
Figure 2. The 2nd-order tetractys character of the first 10
separate polygons.
geometry of the three absent polygons in the
simplest possible way. The seven separate polygons of the inner Tree of Life have
48 sectors
with (48+7=55)
corners, where 55 is the tenth triangular number:
1 + 2 + 3 + 4 + 5 + 6 + 7 + 8
+ 9 + 10 = 55.
Figure 2 shows the first 10 polygons
arranged in a tetractys, the numbers denoting their corners. As they are analogous to the
three Sephiroth of the Supernal Triad, the three absent polygons are located at the
corners of the tetractys. The dodecagon
occupies its centre because, as the last of the polygons making up the inner Tree of Life,
it corresponds to Malkuth, the final Sephirah of Construction. The two triplets of
polygons: (triangle, square, pentagon) & (hexagon, octagon, decagon) correspond to the
two triads of
3
Sephiroth of Construction: Chesed-Geburah-Tiphareth
& Netzach-Hod-Yesod (Fig. 3). The 10 separate polygons have
(27+48=75) sectors with (30+55=85) corners. This is the
number of yods making up the 10 tetractyses in a 2nd-order tetractys. Moreover, the
tetractyses at its three corners contain 30 yods, the seven other tetractyses containing 55 yods. The former are analogous to
the 30 corners of the 27 sectors of the three absent polygons and the latter are analogous
to the 55 corners of the 48 sectors of the seven polygons in the inner
Tree of
Life.
 Figure 3. The two
triads of Sephiroth of Construction. Figure 3. The two
triads of Sephiroth of Construction.
The
white centres of the three corner tetractyses correspond to the centres of the three absent
polygons, their 27 blue yods correspond to the 27 corners of the latter, the seven black
centres of the seven coloured tetractyses correspond to the centres of the seven polygons
and their 48 red yods correspond to the 48 corners of these
polygons (the six red corners of tetractyses correspond to the six corners of the hexagon
and the 42 red hexagonal yods correspond to the 42 corners of the six other polygons). We
see from these detailed correspondences that the 2nd-order tetractys symbolizes the first
10 polygons, with that part of it corresponding to the Supernal Triad symbolizing the three
absent polygons and that part corresponding to the seven Sephiroth of Construction
symbolizing the seven types of polygons in the inner Tree of Life. Here is mathematical
confirmation that an analogy between the 10 Sephiroth of the Tree of Life and the first 10
types of polygons does, indeed, exist; there are far too many correspondences to be
attributable, plausibly, to chance.
Tabulated below are the
geometrical compositions of the 10 Type A polygons, the three absent Type A polygons and
the seven Type A polygons of the inner Tree of Life (numbers in brackets refer to both
sets):
Table 1. Geometrical composition of the 3,
7 & first 10 polygons.
|
|
10
polygons
|
3 absent
polygons
|
7
polygons
|
|
|
separate
|
enfolded
|
separate
|
enfolded
|
separate
|
enfolded
|
|
Number
of corners of sectors (C)
|
85
(170)
|
65 (128)
|
30
(60)
|
26 (50)
|
55
(110)
|
41
(80)
|
|
Number
of sides of sectors (S)
|
150
(300)
|
139
(277)
|
54
(108)
|
52
(103)
|
96
(192)
|
88
(175)
|
|
Number
of sectors (T)
|
75
(150)
|
74
(148)
|
27
(54)
|
27
(54)
|
48 (96)
|
47
(94)
|
|
Number
of geometrical elements
|
310
(620)
|
278
(553)
|
111
(222)
|
105
(207)
|
199
(398)
|
176
(349)
|
|
Comments
1. The Godname EL of Chesed
with number value 31 prescribes the
geometrical composition of the 10 separate polygons because they have 310
(=31×10) corners, sides & triangles. Both sets of 10 separate
polygons have 620 geometrical elements. 620 is the number of
Kether ("Crown") (Fig. 4).
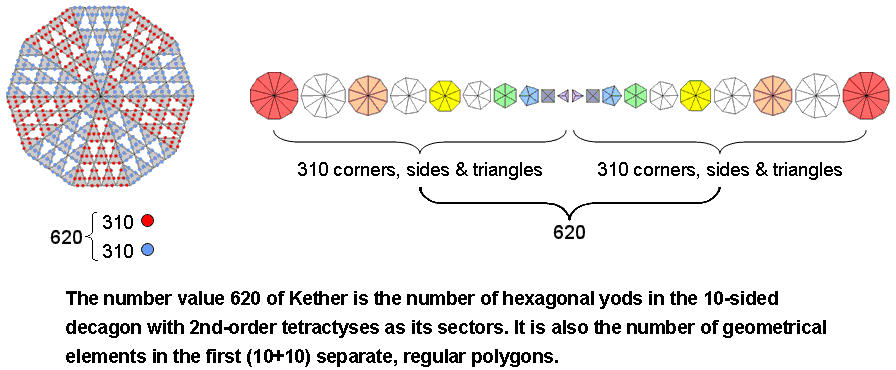
Figure 4
2. The first (10+10) enfolded polygons have 553 geometrical elements, i.e.,
550 elements outside their shared side. This is remarkable for two reasons: 1. 550 =
10(1+2+3+4+5+6+7+8+9+10), i.e., the Decad determines the geometrical composition of
the first (10+10) enfolded polygons; 2. the number of SLs in the Cosmic Tree of Life
is 550 (see here). Embodied, therefore, in the
geometrical composition of the first (10+10) enfolded polygons is CTOL! It is the
polygonal counterpart of the 550 geometrical elements in the faces of the five Platonic
solids (see here);
3. Figure 5 shows the 74
sectors of the first 10 enfolded polygons (the sectors of the three absent polygons have
black sides). They have 65 corners, where
65 is the number value of ADONAI,
the
4
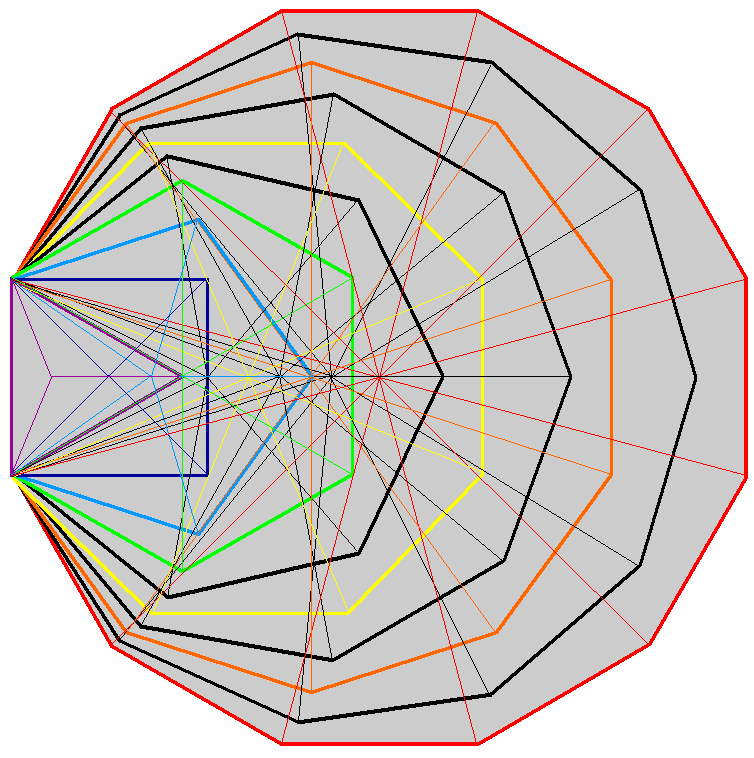 Figure 5. The 74 sectors of the first 10 enfolded
polygons.
Figure 5. The 74 sectors of the first 10 enfolded
polygons.
Godname of Malkuth, and the sum of the first 10 integers after
1:
| |
|
|
2 |
|
| |
|
3
|
4
|
|
|
65 =
|
|
5 |
6 7 |
|
| |
8
|
9
|
10
|
11 |
ADONAI prescribes the
lowest 10 overlapping Trees of Life because they have 65 SLs (see here), so it is highly appropriate
that it should determine the geometrical form of the first 10 enfolded polygons. They also have 65 sides outside their
shared side. Both sets of 10 enfolded polygons have (65+1+65
=131) sides,
where 131 is the number value of Samael, the Archangel of
Geburah. The sectors of the 10 enfolded polygons have 139 sides. Remarkably, this is
the number of yods in the first five enfolded polygons of the inner Tree of Life
(Fig. 6).
|
|

|
|
Figure
6. The
first 5 enfolded polygons
of the inner Tree of Life have 139 yods.
|
Figure 7. The sum of the
integers on the rising edges of the Tetrahedral Lambda is the number
of
corners of internal triangles creating edges of the two polyhedra in the
Polyhedral Tree of Life.
|
The first 10 enfolded
polygons are shaped
by 138 straight lines extending on
either side of their shared side. This number is embodied in
the Tetrahedral
Lambda as the sum
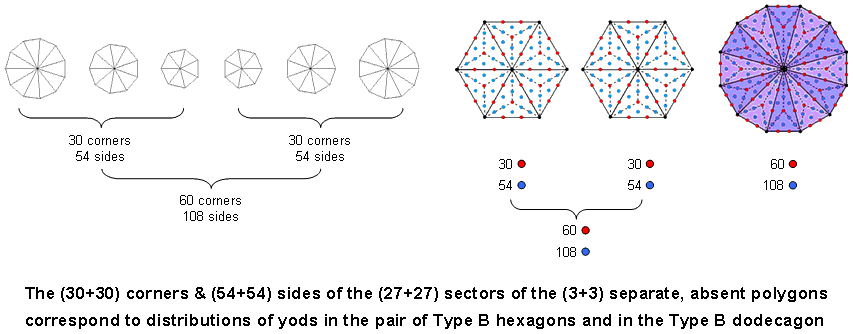
Figure 8.
of the 10
integers
5
(coloured red) arranged on the three
sloping edges of the tetrahedral array of 20 integers (Fig. 7). (Integers at centres of
faces are omitted for the sake of clarity). If the vertices of the two polyhedra making
up the Polyhedral Tree of
Life are joined to their
centres, their resulting 396 internal triangles have 138 corners. Two sides of each hexagon in the inner Tree of
Life are sides of triangles in the outer Tree of Life, forming their side pillars, so
that the first 10 enfolded polygons have 74 sectors with 137 sides that are intrinsic to
them. Here is another way in which they embody the fine-structure number
137.
4. The 27 sectors of the three separate, absent polygons have (30+54=84)
corners & sides, so that the 54 sectors in both sets of these polygons have
168 corners & sides (Fig. 8). Their
shapes are determined by the number of Cholem Yesodoth, the Mundane Chakra of Malkuth. Here is how the
geometry of the three absent polygons embody the number that determines the structure
of each of the 10 toroidal vortices making up a subquark superstring, namely, the
168 turns in one revolution of a helical
whorl of the UPA. It is so plain and intuitively natural that it leaves no doubt
about the archetypal nature of this geometry. It is reproduced in the pair of
separate Type B hexagons and in the Type B dodecagon as the (84+84=168) yods needed to transform their sectors into Type A
triangles. Their (30+30) corners are symbolised by the (30+30) red yods in the
tetractys sectors and their (54+54) sides are symbolised by the (54+54) blue yods
added by converting the sectors into Type A triangles. Enfolded, the (3+3) separate,
absent polygons have (50+103=153)
corners & sides, where 153 is the
number value of ELOHIM SABAOTH. The Godname ELOHIM with number value 50 prescribes their 50 corners and SABAOTH with number value 103 prescribes their 103 sides. The division of these 50 corners into the two endpoints of the shared side and
the (24+24=48) corners outside it is
characteristic of holistic systems (see here);
5. The (3+3) separate, absent polygons have 222 geometrical elements.
This is the number of hexagonal yods associated with each set of seven enfolded polygons
when their 47 sectors are tetractyses (Fig. 9).
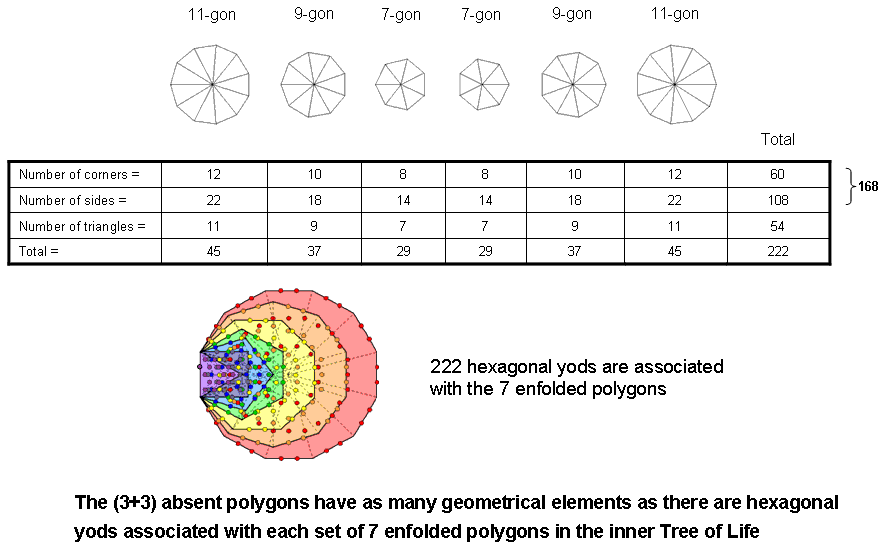
Figure 9. The (3+3) absent polygons have as
many geometrical elements as there are hexagonal yods associated with each set of 7
enfolded polygons in the inner Tree of Life.
(See also #12). It is also the number of permutations of the rows of yods in the
three orientations of a tetractys (see here). Notice that each orientation generates 74 permutations of the three
rows of yods, where 74 is the number of sectors of the 10 enfolded polygons. This is
also the number of vertices in the "144 Polyhedron" (see second diagram in comment
(3)).
6. The 10 enfolded polygons have 55 corners that do not coincide with centres
of polygons, where
55 = 1 + 2 + 3 + 4 + 5 + 6 + 7 + 8 + 9 +
10:
The three enfolded, absent polygons
have 21 corners outside their shared side and the seven enfolded
polygons of the inner Tree of Life have 34 corners that are not also
centres. 21, 34 & 55 are three, successive members of the Fibonacci series
that governs the growth patterns of many living things:
0, 1, 1, 2, 3, 5, 8,
13, 21, 34, 55, 89, 144, ....
See Article 50 (Part 1) & Article 50 (Part 2) for how Fibonacci numbers manifest in various sacred
geometries.
6
Tabulated below in Table 2 are the yod
populations of the 10 polygons, the three absent polygons and the seven polygons of the
inner Tree of Life when constructed from tetractyses (numbers in brackets refer in each
case to both sets):
Table 2. Yod
populations of the 3, 7 & first 10 polygons.
|
|
10 polygons
|
3 absent polygons
|
7 polygons
|
|
|
separate
|
enfolded
|
separate
|
enfolded
|
separate
|
enfolded
|
|
Number
of corners of sectors (C)
|
85
(170)
|
65 (128)
|
30
(60)
|
26
(50)
|
55 (110)
|
41
(80)
|
|
Number
of hexagonal yods = 2S + T
|
375
(750)
|
352
(702)
|
135
(270)
|
131 (260)
|
240
(480)
|
223
(444)
|
|
Number
of yods on sides of tetractyses = C + 2S
|
385
(770)
|
343
(682)
|
138
(276)
|
130
(256)
|
247
(494)
|
217
(430)
|
|
Number
of yods = C + 2S + T
|
460
(920)
|
417
(830)
|
165
(330)
|
157
(310)
|
295
(590)
|
264
(524)
|
|
Comments
1. The 10 enfolded polygons
have 352 hexagonal yods. i.e., 350 hexagonal yods outside their shared side. This is
both the number of hexagonal yods on the sides of the 94 tetractyses making up the (7+7)
enfolded polygons and the sum of the 20 integers in the Tetrahedral Lambda (see Fig. 7
here). There
are 351 hexagonal yods associated with each set of 10 enfolded polygons,
where
351 = 1 + 2 + 3 +... + 26
is the number value of Ashim, the Order of
Angels assigned to Malkuth. This shows in a very direct, unmistakable way how YAHWEH, the
Godname of Chokmah with number value 26, prescribes the hexagonal yod population of the 10
enfolded polygons. It also prescribes the 26
corners of the three enfolded, absent polygons. Indeed, there are 73 yods on their sides, where 73 is the number of
Chokmah (Fig. 10). This is the number of yods in the 12 tetractyses needed to
construct the Star of David
(Fig.
|

|
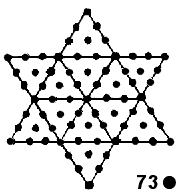 |
|
Figure 10.
|
Figure
11. The Star of David representation of the number 73 of Chokmah (“Wisdom”).
|
11).
Furthermore, YAHWEH prescribes the hexagonal yod population of the (3+3) enfolded,
absent polygons, which have 260 (=26×10) hexagonal yods. The
three enfolded, absent polygons have 131 hexagonal yods,
where 131 is the number value of Samael, the Archangel of
Geburah. 129 hexagonal yods are outside their shared side,
where 129 is the number value of YAHWEH SABAOTH, the Godname of
Netzach. Table 2 indicates that there are
702 hexagonal yods on the
sides of the
tetractyses in
the
 Figure 12. 350 hexagonal yods line the (47+47) tetractyses in the
(7+7) enfolded polygons. Figure 12. 350 hexagonal yods line the (47+47) tetractyses in the
(7+7) enfolded polygons.
(10+10)
enfolded polygons. 350 (=35×10)
hexagonal yods are distributed on either side of the root edge. This 35:35 division is
characteristic of holistic systems, e.g., the 70 yods of the Tree of Life are composed
of 35 yods in its trunk and 35 yods in its branches (see here). Amazingly, the
(7+7) enfolded Type A polygons have 350 hexagonal yods on the sides of their 94
tetractyses (Fig. 12). This demonstrates once again the holistic character of the
(10+10) polygons;
2. There are 385 yods on the sides of the 75 tetractys sectors of the first 10
separate polygons, where
7
385 = 12 +
22 + 32 +
42 + 52 +
62 + 72 +
82 + 92 +
102.
(Fig. 13). 138 yods line the 54
sides of the 27 tetractys sectors of the three separate, absent polygons. Compare this
with the fact discussed in (3) above that the 74 sectors
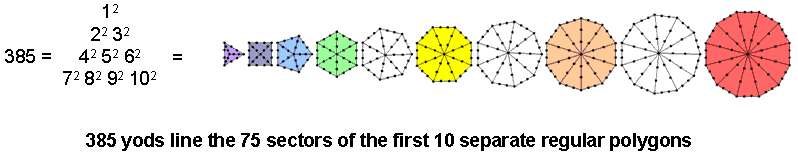
Figure 13. 385 yods line the 75 sectors of
the first 10 separate, regular polygons.
of the 10 enfolded
polygons have 138 sides outside their shared side. The same number measures the yods in
two joined, Type A dodecagons outside their
shared side. The Tetrad (4) determines the 256 yods lining
the 54 tetractyses in the (3+3) enfolded, absent polygons because 256 =
44;
3. The first 10 separate polygons contain 460 (=46×10) yods, where 46 is the number of
yods in a Type B Triangle. As the average yod population of the first 10 polygons,
the number 46 is well-known as
the number of chromosomes in
the human cell. The first (10+10) separate polygons contain 920 yods, so that 900
(=90×10) yods surround their centres. The number 90 is the sum of the 10 integers in
the Lambda Tetractys and is shown in many analyses on the author’s website to be
embodied in sacred geometries. Its manifestation here is no coincidence, of course,
because of the holistic nature of the first 10 polygons. The three separate, absent
polygons contain 165 yods, where
165 =
12 + 32 +
52 + 72 +
92.
The three enfolded, absent polygons contain 157
yods, i.e., 153 yods outside their shared
side, where 153 is the number value of
ELOHIM SABAOTH, the Godname of Hod.
The (3+3) enfolded, absent polygons contain 310 (=31×10) yods, where 31 is the number value of EL, the Godname of Chesed.
How appropriate that this Godname, which
translates as "God," prescribes the yod population of those polygons which correspond to
the Supernal Triad, the triple Godhead! How also appropriate
that 155 yods are associated with each set of three polygons,
for 155 is the number value of ADONAI MELEKH, the complete Godname of Malkuth!
This number, truly, quantifies the form of the three absent polygons. Its geometrical
representation is discussed here.
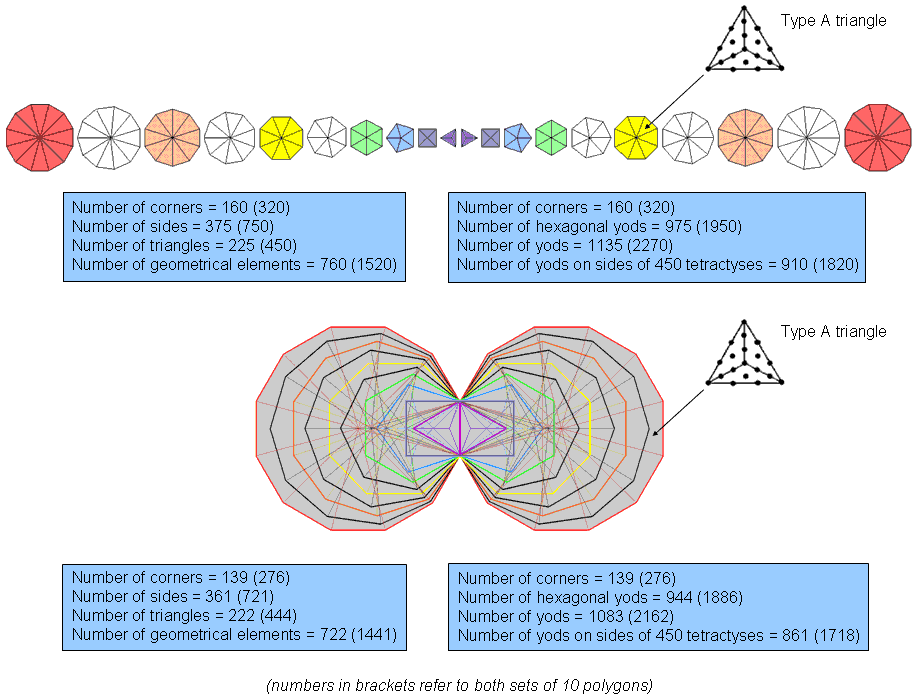
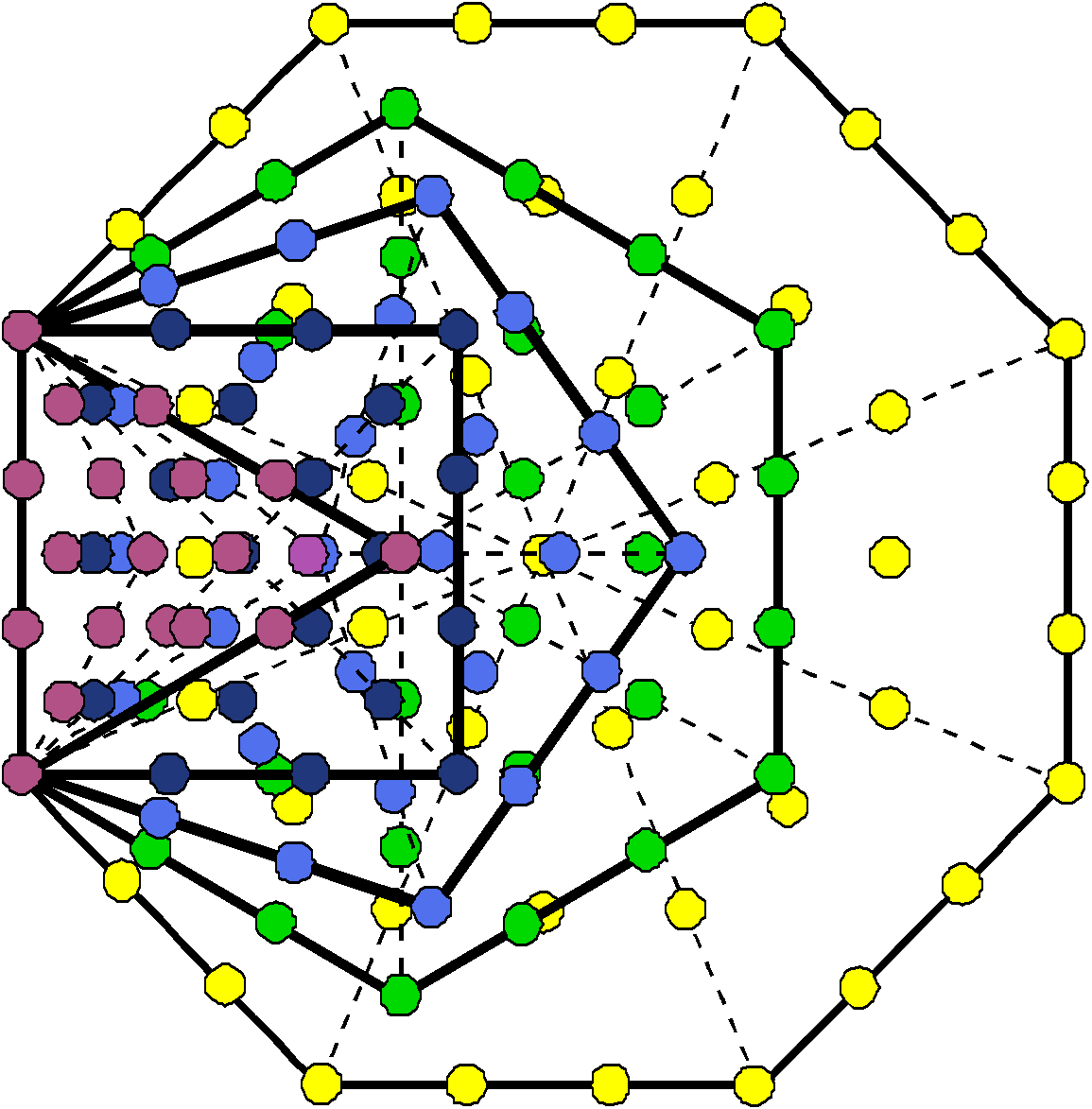
Figure 14. Properties of the first (10+10) Type B
polygons.
Now consider the case
where all the polygons are Type B (Fig. 14). Tabulated below in Table 3 are the
geometrical compositions of the first 10 Type B polygons, the three absent Type B
polygons and the seven
8
Type B polygons of the inner Tree of Life (numbers in brackets refer to
both sets):
Table 3. Geometrical
composition of the 3, 7 & first 10 Type B polygons.
|
|
10 polygons
|
3 absent polygons
|
7 polygons
|
|
|
separate
|
enfolded
|
separate
|
enfolded
|
separate
|
enfolded
|
|
Number
of corners of sectors (C)
|
160
(320)
|
139
(276)
|
57
(114)
|
53
(104)
|
103 (206)
|
88
(174)
|
|
Number
of sides of sectors (S)
|
375
(750)
|
361
(721)
|
135
(270)
|
133
(265)
|
240
(480)
|
229
(457)
|
|
Number
of sectors (T)
|
225
(450)
|
222
(444)
|
81
(162)
|
81
(162)
|
144
(288)
|
141
(282)
|
|
Number
of geometrical elements
|
760
(1520)
|
722
(1441)
|
273
(546)
|
267
(531)
|
487
(974)
|
458
(913)
|
|
Comments
1. The 10 separate polygons have 760
(=76×10) geometrical elements. They are
prescribed by YAHWEH ELOHIM, the Godname of Tiphareth with number value
76. (760−30=730=73×10) geometrical elements are outside the sides
that become shared when they are enfolded. The 10 enfolded polygons have 139 corners,
i.e., 137 corners outside their shared side determine their shapes, where 137 is the
number whose reciprocal is approximately the fine-structure constant. This
number is embodied in the (5+5) enfolded polygons shown in Figure 6 as the 137 yods
associated with each set. Alternatively, as the topmost corner
of the hexagon coincides with the lowest corner of the hexagon enfolded in the next
higher Tree of Life, 137 corners intrinsic to the 10 enfolded polygons
are associated with each set of 10 enfolded polygons. The latter are displayed below in
Figure 15 (notice there is no green yod at the top of the two
hexagons):
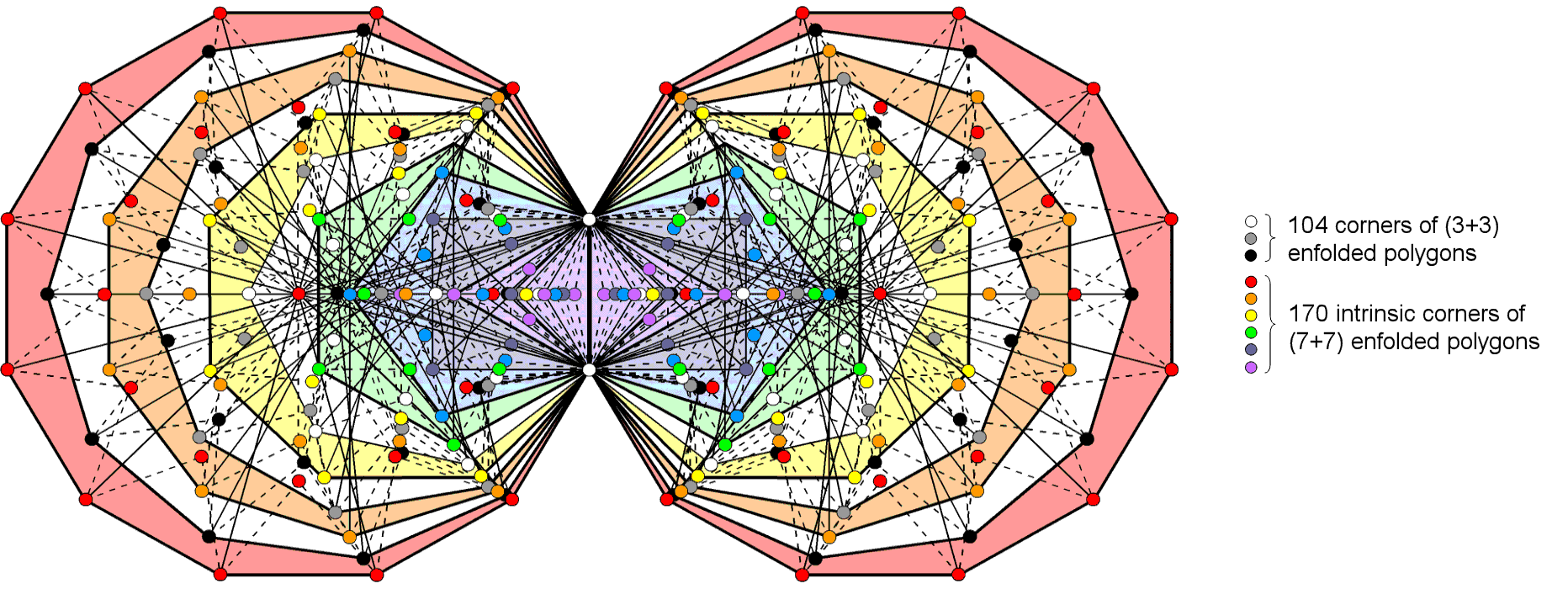
Figure 15. 137
corners are intrinsic to each set of 10 enfolded polygons. 137 is the 33rd prime number,
where 33 = 1! + 21 + 3! + 4!.
Enfolded, they have 361 sides, where 361 = 192, i.e., the square of the tenth odd integer. ELOHA, the Godname of Geburah with
number value 36, determines their 360
(=36×10) sides
outside their shared side;
2. The (10+10) enfolded polygons have 444 triangles. This is the number of
hexagonal yods in the (7+7) enfolded Type A polygons (see last diagram here). Symbolised,
therefore, in the inner form of the Tree of Life are the number of triangular sectors in
the (10+10) enfolded Type B polygons;
3. The three enfolded polygons have 267 geometrical elements, i.e., 264
elements outside their shared side. This is the number of yods in the seven enfolded
Type A polygons;
4. The 10 enfolded polygons have 500 (=50×10) corners & sides. ELOHIM, the Godname of Binah
with number value 50, prescribes their
shape. As their shared side consists of two endpoints and one line, there are 497
corners & sides outside the root edge. In the context of overlapping Trees of
Life where each Tree has its own set of (7+7) enfolded polygons, if we now consider
the hidden (3+3) polygons accompanying each set, there are 496 geometrical elements that are intrinsic to each set
of 10 enfolded polygons because the topmost corner of the hexagon coincides with the
lowest corner of the hexagon enfolded in the next higher Tree of Life and so is
shared with the polygons enfolded in this Tree. This number is the number value of Malkuth.
Embodied in the 10 enfolded polygons is the dimension of SO(32) and
E8×E8, the two gauge symmetry groups that permit
interactions between superstrings that are free of quantum anomalies;
5. As the three enfolded
polygons have 26 corners, there are
(500−26=474) corners & sides other than these in the 10
enfolded polygons. 474 is the number of
Daath (“knowledge”).
Tabulated below in Table 4 are the yod
populations of the first 10 Type B polygons, the three absent Type B polygons and the
seven Type B polygons of the inner Tree of Life (numbers in brackets refer in each case
to both sets of polygons):
Table 4. Yod populations of the 3, 7 & first 10
polygons.
|
|
10 polygons
|
3 absent polygons
|
7 polygons
|
|
|
separate
|
enfolded
|
separate
|
enfolded
|
separate
|
enfolded
|
|
Number
of corners of tetractyses = C
|
160
(320)
|
139
(276)
|
57
(114)
|
53
(104)
|
103 (206)
|
88
(174)
|
|
Number
of hexagonal yods = 2S + T
|
975
(1950)
|
944
(1886)
|
351 (702)
|
347
(692)
|
624
(1248)
|
599
(1196)
|
|
Number
of yods on sides of tetractyses = C + 2S
|
910
(1820)
|
861
(1718)
|
327
(654)
|
319
(634)
|
583
(1166)
|
546
(1088)
|
|
Number
of yods = C + 2S + T
|
1135
(2270)
|
1083
(2162)
|
408
(816)
|
400
(796)
|
727
(1454)
|
687
(1370)
|
|
Comments
1. YAHWEH with number value 26 prescribes
the 351 hexagonal yods in the three separate, absent polygons
because
351 = 1 + 2 + 3 + .... +
26;
2. There are 910 (=91×10) yods on the sides of
the 225 tetractyses in the 10 separate polygons. Embodied in the number quantifying
the boundaries of their tetractyses is the number (91) of Trees of Life in
the Cosmic Tree of
Life. The (10+10)
separate polygons have 1820 such yods on the boundaries of their 450 tetractyses.
Remarkably, this is the number of yods surrounding the centres of the five Platonic
solids when their faces are constructed from tetractyses and their interior triangles
meeting at their centres are Type A (see Table 1 in Article 3);
9
3. The number of yods in the (10+10) separate polygons = 2270 =
227×10. This shows how EL ChAI, the Godname of Yesod with number
value 49, prescribes the (10+10) separate polygons, as 227 is
the 49th prime number;
4. The number of yods in the (10+10) enfolded polygons = 2162 =
2×1081. Amazingly, the number value 1081 of Tiphareth
("Beauty") is the number of yods associated with each set of the first 10 enfolded
polygons! As
1081 = 1 + 2 + 3 + ... +
46,
the simplest Type B polygon
— the triangle — embodies this number arithmetically because it has 46 yods. It is
remarkable that the number of Tiphareth should measure the yods associated with the 10
enfolded polygons, whilst the number of its Godname should measure the number of points,
lines & triangles in the 10 separate polygons. It would be tantamount to believing
in miracles to dismiss this as a coincidence. Instead, this property is an eloquent
expression of the beautiful power and intelligence of genuine sacred geometries. It
reveals the way in which the true meaning of the word 'sacred' transcends all cultural
contexts, whatever religion in which these geometries originate (and even if it was
inspired by no religion). According to
Table 4, there are 1088 yods on the sides of the 282 tetractyses in the (7+7) enfolded polygons. The topmost
corners of the two hexagons coincide with the lowest corners of the hexagons enfolded in
the next higher Tree of Life. Hence, 1086 boundary yods are intrinsic to each set of
(7+7) enfolded polygons, i.e., 1082 intrinsic, boundary yods are outside the root edge.
1082 is the 1081st integer after 1, once
again showing how the number of Tiphareth determines the shape of the inner Tree of
Life;
5. The first 10 enfolded Type B polygons have 222 tetractys sectors
with 139 corners, i.e., 137 corners outside their root
edge. The number determining the
fine-structure constant shapes the first 10 enfolded Type B polygons as the number of
points marking out their corners outside their shared side. This property is analogous to the 139 yods in the first five
enfolded polygons shown in Figure 6;
6. The first 10 enfolded polygons have 944
hexagonal yods. 222 of them are at the centres of the 222 tetractyses, leaving 722 hexagonal yods
lining their sides. 720 of these are outside the root edge. Hence, (137+720=857) such
yods line their sides. Including the four yods of the root edge, (4+857=861) yods
line their sides. Surrounding the 10
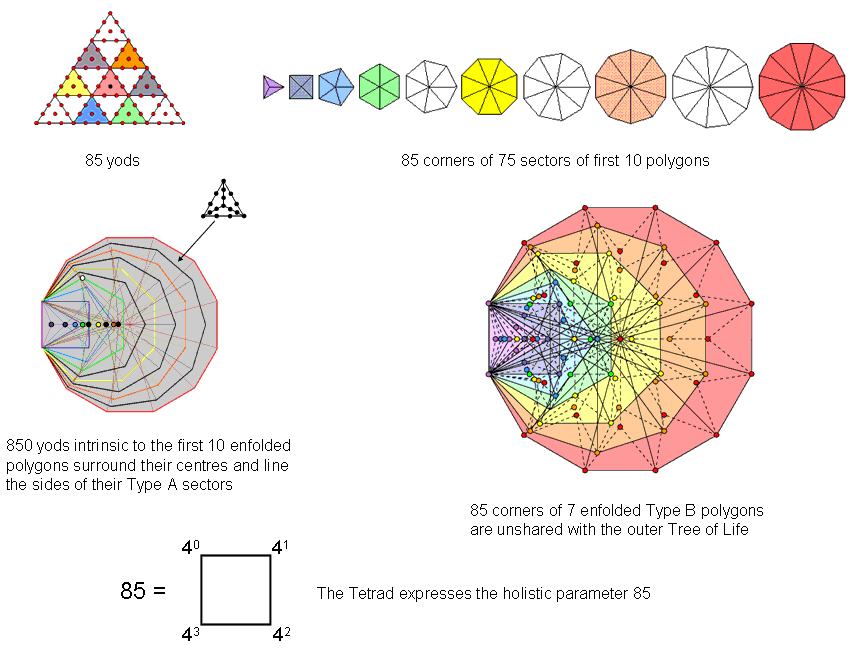
Figure 16. The first 10 enfolded Type B
polygons embody the holistic parameter 85.
centres of the polygons
are 851 boundary yods. One of them (indicated by a white yod in Figure 15) is the topmost
corner of the hexagon, which coincides with the lowest corner of the hexagon enfolded in
the next higher Tree of Life. This is the only corner which is shared with polygons
enfolded in the next higher Tree, so that 850 (=85×10) boundary yods intrinsic to
the 10 polygons enfolded in any Tree of Life surround their centres (Fig. 16). This
demonstration that they embody the holistic parameter 85, which was encountered earlier as
the 85 corners of the first 10 separate polygons (see Fig. 2) is further evidence of their
holistic character;
7. Of the 857 yods outside the root edge that
line sides of 222 tetractyses, 24 yods are corners of the 27 sectors of the three
types of polygons absent from the inner Tree of Life. (857−24=833) yods other than these corners line the sides of the
tetractyses making up the 10 enfolded Type B polygons. 833 is the number value of Chaioth ha Qadesh (“Holy Living Creatures”), the Order
of Angels assigned to Kether;
8. Of the 857 boundary yods outside the root edge,
21 yods are corners of the three enfolded
polygons,
10
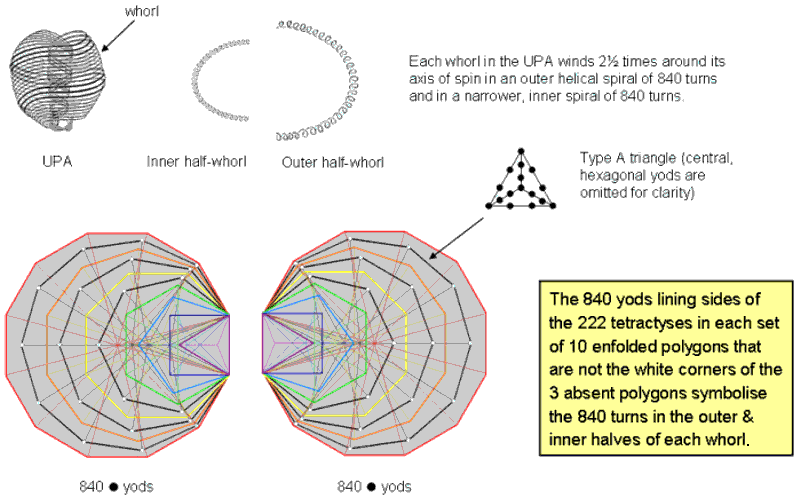
Figure 17. The 840
yods lining tetractyses in the 10 enfolded Type B polygons that
are not corners of the three absent polygons
symbolize the 840 turns in the outer & inner halves of
each whorl of the UPA/subquark
superstring.
where 21 is the number value of EHYEH, the Godname of Kether.
(857−21=836) yods outside the
root edge line tetractyses that are not corners of the three polygons that were
hypothesized at the beginning of this article to correspond to Kether, Chokmah &
Binah. Including the root edge, (836+4=840) yods other than these corners line sides
of 222 tetractyses (Fig. 17). Embodied in the 10 enfolded polygons is the superstring
structural parameter 840 — the number of turns in an outer or inner half of each
whorl of the UPA remote-viewed by Annie Besant & C.W. Leadbeater over a century
ago and identified by the author as the subquark state of the E8×E8 heterotic superstring (see his books available on
his website). This property is the full expression of the 84 corners & sides of
the three separate Type A polygons that are absent from the inner Tree of Life, as
shown in Figure 8. Notice that the (21+21=42)
corners of the (3+3) enfolded polygons outside their shared side play no part in
determining this number. It is the geometrical realization of the fundamental,
metaphysical dichotomy between the Supernal Triad, which exist outside all levels of
reality, and the seven Sephiroth of Construction, which express these realities
(actually, the seven cosmic planes of consciousness mapped by the Cosmic Tree of
Life);
9. The (10+10) enfolded polygons contain 1886
hexagonal yods. According to Table 1, the 54 sectors of the (3+3) enfolded polygons have
103 sides, where 103 is the number of SABAOTH (“Hosts”), part of the
Godnames of Hod & Netzach. 206 hexagonal yods line the sides of their sectors.
Hence, (1886−
206=1680) extra hexagonal yods are
needed to turn the 148 sectors of the
(10+10) enfolded Type A polygons into the 444 tetractyses making up the (10+10) Type
B polygons. 1680 yods line the sides of the two separate sets of 10 enfolded Type B
polygons and 1680 hexagonal yods (840 hexagonal yods in each set) have to added to
the (3+3) enfolded Type A polygons in order to generate the (10+10) enfolded Type B
polygons. This reveals how they embody the superstring structural parameter 1680.
There are (148+10+10=168) corners inside the
boundaries of all the polygons, showing how they embody the number
of CholemYesodoth, the Mundane
Chakra of Malkuth. The following, remarkable relationship
emerges:
11
1886 hexagonal yods = 206 hexagonal yods + 1680
hexagonal yods.
The human skeleton has 206
bones and a helical whorl of the UPA/superstring has 1680 turns. It is, of course,
meaningless to add together such different entities as bones and polarised oscillations
of a vortex
ring — a component of the superstring.
However, this is not the point. It cannot be regarded as merely a curious coincidence,
for both numbers have definite geometrical meanings in the context of
the geometry of the first 10 polygons, namely, 206 hexagonal yods lining
sides of the 54 sectors of the
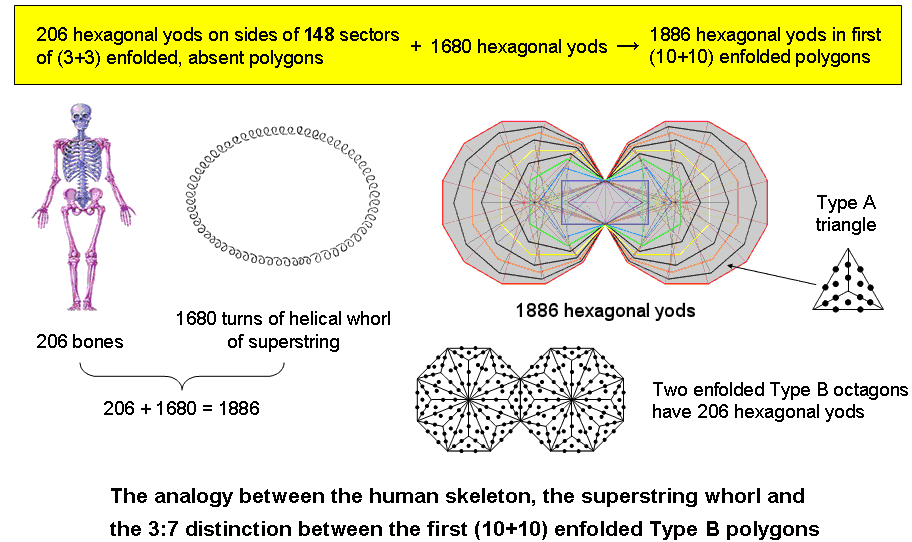
Figure 18.
The analogy between the human
skeleton, the superstring whorl and the 3:7 distinction between the first (10+10)
enfolded Type B polygons.
(3+3) enfolded Type
A polygons absent from the
inner Tree of Life and 1680 hexagonal yods added by the conversion of the (10+10)
enfolded polygons into Type B polygons (Fig. 18). The division stems from the basic
distinction between the Supernal Triad (the triple Godhead) and the seven Sephiroth
of Construction. What is remarkable is that the primary, structural parameters of the
human body and the whorl should add up to the number of hexagonal yods in the (10+10)
enfolded polygons! Holistic parameters can be composed of several other holistic
parameters, each having its own context that is not necessarily connected to one
another. The number 206 is also embodied in the two enfolded, Type B octagons because
they contain 206 hexagonal yods. This means that the other (9+9) enfolded polygons
contain 1680 hexagonal yods, each set of nine polygons having 840 hexagonal yods
outside their shared, root edge. As a Type A octagon contains
49 yods (see here), EL ChAI, the Godname of Yesod with number value
49,
can be said to prescribe the number of circularly polarised oscillations in the
vortex ring, or toroidal vortex, that is each whorl of the UPA/subquark
superstring.
12
|