|
by Stephen M. Phillips Website: http://smphillips.mysite.com
“In ancient times, music was something other than mere pleasure for the ear: it was like an algebra of metaphysical abstractions, knowledge of which was given only to initiates, but by the principles of which the masses were instinctively and unconsciously influenced. This is what made music one of the most powerful instruments of moral education, as Kong-Tsee (Confucius) had said many centuries before Plato.” G. de Mengel, Voile d’Isis Abstract
1 1. The tetrahedral Platonic Lambda
The tetrahedron of 20 numbers has the following musical virtue: the extended Lambda
* This is not intended as a criticism of Plato, who may have known about the tetrahedral generalisation.
2 tetractys generates the
tone ratios of octaves along one side and perfect fifths along another side. But the numbers
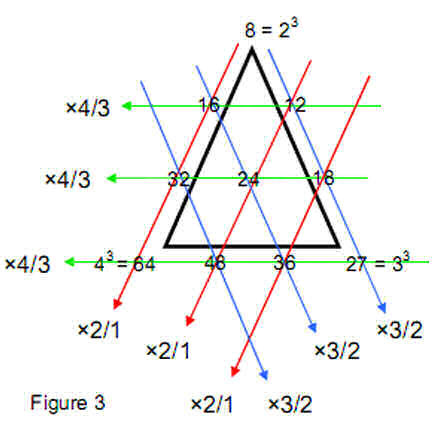
starting with 6 and The number 24 (=1×2×3×4) is at the centre of the fourth face*. Figure 4 displays the lattice of tone ratios, starting with 1, the fundamental, that are created by dividing every number in the tetractys and outside it by 24. Using any other number in or outside the tetractys as divisor would have created the same lattice of tone ratios. Overtones are shown in yellow circles, red lines connect octaves (×2), green lines connect perfect
* 6, the centre of the Lambda tetractys, is the fourth overtone and 24 is the tenth overtone. Integers 6, 8, 12 and 24 at the centres of the four faces have the ratios 1, 3/2, 4/3, 2, 3 and 4 of the integers 1, 2, 3 and 4. 3 Figure 4 4 fourths (×4/3) and blue lines connect perfect fifths (×3/2). The tone interval of 9/8 is also indicated by the orange line joining the centre of the tetractys (coloured grey) to one corner. The tone ratios 27/16 of note A and 243/128 of note B are similarly defined by, respectively, indigo and violet diagonals extending from the number 1 to corners of larger triangles. Successive notes of the scale for each octave are joined by dashed lines. They zigzag between an octave, the seventh note of the octave and its perfect fourth, i.e., Table of tone ratios of eleven octaves of the Pythagorean scale
(Red cells enclose integer notes up to end of tenth octave) between the extremities of the Pythagorean scale and its midpoint. 2. The first ten octaves The tone ratios of the 71 notes in the first ten octaves are shown in the table above (red cells contain overtones and blue cells enclose notes beyond the tenth octave). The last column lists as a running total the number of overtones of the fundamental with a tone ratio of 1. 5 Comments
and 128 is the 21 st overtone and the 50 th note (the last note of the seventh octave). This shows how Ehyeh, Yahweh and Elohim, the Godnames of the Supernal Triad, prescribe the range of pitch between the first seven notes (1). 256, which is part of the leimma 256/243 between notes E and F and between B and C of the next octave, is the 36th note, counting from the beginning of the fourth octave. This shows how Eloha, Godname of Geburah with number value 36, defines the ‘leftover’ between adjacent octaves. The 36 th note after 1 has a tone ratio of 36 ;
are integers and 31 are fractions. This shows how the Godname El of Chesed with number value 31 determines the number of notes whose tone ratios are not whole numbers, whilst Eloha prescribes the number of notes because 71 is the 36 th odd integer. It also demonstrates how the Pythagorean Tetrad (4) defines the number of integer tone ratios in ten octaves. The 71st note has a tone ratio of 1024 = 210. This is the smallest number with ten prime factors (all 2), showing the Pythagorean character of the last note of the tenth octave — the 40th note that is an integer;
* The number values of the Sephiroth, their Godnames, Archangels, Angels and Mundane Chakras are written throughout the text in boldface. 6 Hence, 972 = 33 + 63 + 93. This property is an example of the beautiful, mathematical properties of the first ten octaves (the reason for this will be given shortly). As 2700 = 33 + 63 + 93 + 123 and 100 = 13 + 23 + 33 + 43, the largest integer 27 can be expressed as the ratio:
Once again, it is the Pythagorean Tetrad that expresses a number important to the mathematics of the Pythagorean scale, for both the numerator and the denominator in the ratio are the sum of four cubes. It was pointed out in Article 11 that Yahweh prescribes the number 243 in the leimma because it is the 26th overtone. 243 = 33 + 63, i.e., it is the sum of the first two of the three cubes summing to the value of the tone ratio of the seventh note of the tenth octave. 3 and 6 are the integers in 36, the number value of Eloha. The table indicates that 63 = 216 is the tone ratio next smaller than 243. This is the number of Geburah whose Godname defines the number 256, as indicated in comment (1), as well as the number 243. Because the tone ratios of
corresponding notes in n successive octaves all increase by the same factor of 2, there are
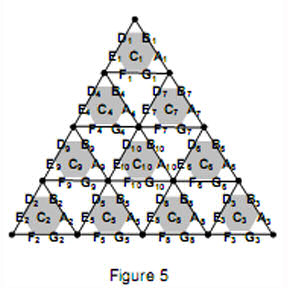
as many overtones Let us now represent the 70 notes of the ten octaves by what the author has called in previous articles a ‘2nd-order tetractys’ (Fig. 5). The 21 notes of the first three octaves
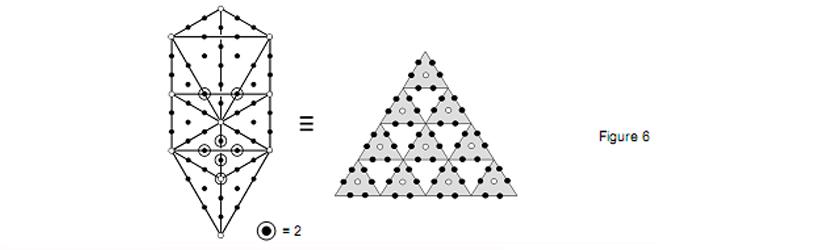
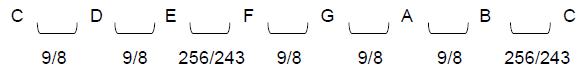
are arranged at the corners and centres of hexagons at its three corners and the 49 notes of the next seven octaves are at the corners and centres of seven hexagons arranged at the corners and centre of a larger hexagon (2). The ten tonics Cn (1≤n≤10) are at the centres of the hexagons. The centre of the 2nd-order tetractys denotes the tonic of the tenth octave with tone ratio 512 (C10). This kind of tetractys is a more differentiated version of the Pythagorean symbol of divine wholeness. It is why the 70th number, 972, exhibits arithmetic properties typical of the beautiful harmonies manifested by this pattern. Figure 6 displays the equivalence between the 2nd-order tetractys* and the Tree of Life with its 16 triangles turned into tetractyses. The ten corners of these triangles correspond to the centres of the ten tetractyses (both shown as white circles). The tonics of the ten octaves can be assigned to the positions of the ten Sephiroth and the remaining 60 notes assigned to the 60 black yods. The first seven notes of each octave formally correspond to a Sephirah. This is the reason for our considering the first ten octaves of the Pythagorean scale. The mathematical beauty of this Tree of Life pattern has already begun to show itself in the properties of the 70th note discussed above. Let us now consider the integer tones in the ten octaves. As pointed out in comment 2 above, their number 40 can be represented as a tetractys array of the number 4. The sum of those 4’s at its corners is 12, leaving 28 as the sum of the seven other 4’s. Yods at corners of a tetractys correspond to the Sephiroth of the Supernal Triad and the seven other yods correspond to the Sephiroth of Construction. The 12:28 division of the 40 integer tone ratios therefore corresponds to the Kabbalistic distinction between the * Actually, it is a slightly different version of that shown in Figure 4. The difference is immaterial. 8 subjective and objective Sephiroth of the Tree of Life. The largest number 27 in Plato’s Lambda is the twelfth such tone ratio (A of the 5th octave). 4 is the fourth integer tone ratio and 12 is the eighth. The former is the 15th note and the latter is the 26 th note. This shows how the Godnames Yah with number value 15 and Yahweh with number value 26 that are assigned to the Sephirah Chokmah mark out notes that correspond to successive members of the Supernal Triad. Yahweh also defines the twelfth integer tone ratio because 27 is the 26th integer after 1 (for the Pythagoreans, 27 would be the 26th true integer because they regarded the number 1 not as an integer but as the source and principle of all numbers). The 40 integer tone ratios comprise 11 octaves (note C) and 29 others. 29 is the 15th odd integer after 1, showing how the Godname Yah with number value 15 defines the number of overtones in ten octaves that are not merely octaves. There are five whole tone intervals (9/8) and two leimmas (256/243) that separate the eight notes of an octave*.
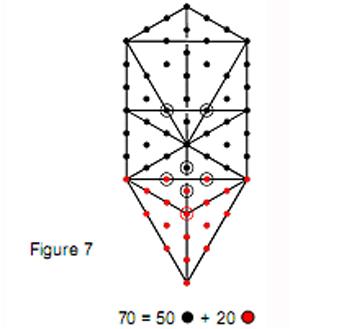
* This 5:2 division corresponds in the Tree of Life to the lowest, five Sephiroth of Construction, which are always shared with overlapping trees, and to Chesed and Geburah, which are unshared. 9 yods lie on n tetrahedra, so that this 5:2 correspondence exists only for a single Tree of Life. Counting from the fundamental of the first octave, there are ten overtones up to the perfect fifth of the fifth octave with tone ratio 24: 2, 3, 4, 6, 8, 9, 12, 16, 18, 24. Of these, four (2, 4, 8, 16) are octaves, leaving six others. This 4:6 division corresponds in the Tree of Life to the four lowest Sephiroth at the corners of a tetrahedron and the uppermost six Sephiroth. The sum of the Godname numbers of the latter is
that is, they sum to the number of yods in 24 tetractyses. This suggests something special about the number 24. This might be suspected, because it is 1×2×3×4, i.e., the number of permutations of four objects, which shows its Pythagorean character.
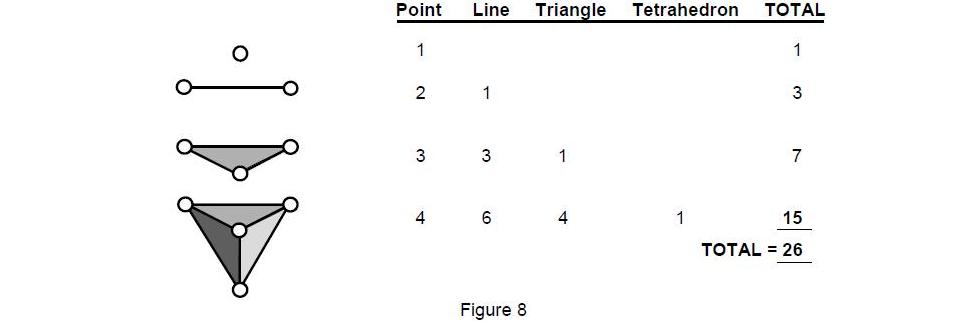
It is also the 26th note and the tenth overtone, both counting from 2, the first overtone. This corresponds in the Tree of Life to the fact that the tenth Sephirah, Malkuth, is, as its lowest point, the 26th and last geometrical element in its trunk (Fig. 8). The significance of the perfect fifth of the fifth octave of the Pythagorean scale is that it is the seventh of a set of perfect fifths, the first five being successive:
(Subscripts denote the octave number) 10 Including the tonic of the first octave, there are 33 notes up to G5, where 33 = 1! + 2! + 3! + 4!, i.e., 33 is the total number of permutations of four rows of 1, 2, 3 and 4 objects arranged in a tetractys. Of these notes, 11 are integers and (33–11=22) are fractional, where 22 = 14 + 23 + 32 + 41. The latter notes comprise 16 notes in the first three octaves and six notes
in the fourth and fifth octaves up to the last fifth. This 6:16 division corresponds in the
Tree of Life to the six Paths that are edges of the tetrahedron whose corners are the lowest
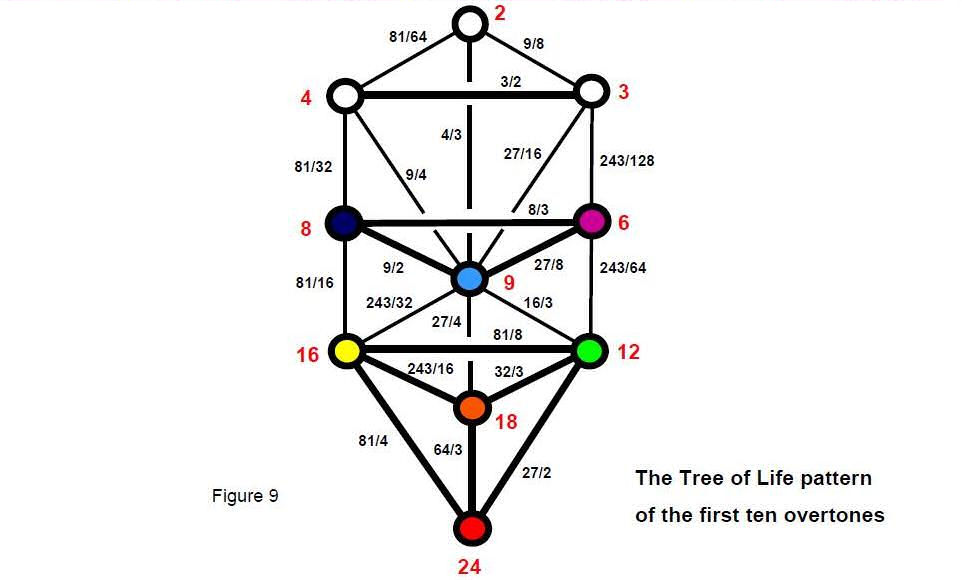
four Sephiroth and the 16 Paths outside it (see Fig. 7). The 32 overtones and notes up to G5 conform to the
geometrical pattern of the Tree of Life, the ten overtones corresponding to the ten
Sephiroth and the 22 fractional notes corresponding to the 22 Paths connecting the Sephiroth
(Fig. 9). The ordering of notes in Figure 9 follows the traditional Kabbalistic (Thick lines are Paths of the trunk of the Tree of Life)
11 numbering of Paths. As the tenth overtone, the tone ratio 24 corresponds to the lowest Sephirah, Malkuth, which signifies the outer, physical form of anything embodying the universal blueprint of the Tree of Life. It is this correspondence that makes the number 24 significant vis-à-vis superstring theory, as will be explained in Section 6. Arranged in a tetractys:
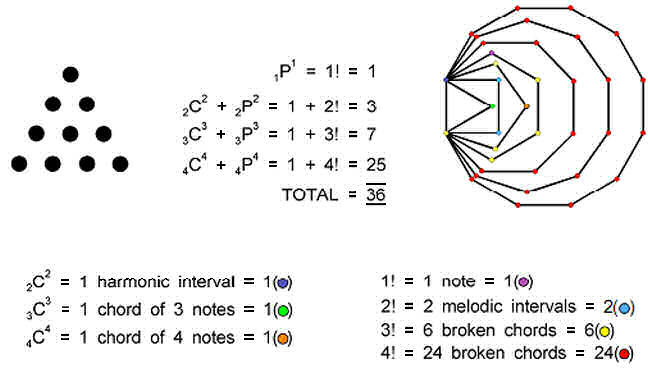
the ten overtones have 16 combinations of two or more notes selected from each row. They comprise ten harmonic intervals, five chords of three notes and one chord of four notes. These correspond in the trunk of the Tree of Life to, respectively, its ten Paths, five triangles each with three corners and the tetrahedron with four corners (see Fig. 9). Alternatively, the 16 harmonic intervals and chords correspond to the 16 triangles of the Tree of Life itself. The ten harmonic intervals correspond to the ten triangles below the level of the path joining Chesed and Geburah, and the six chords correspond to the six triangles either above or projecting beyond this line, which, according to Kabbalah, separates the subjective Supernal Triad from the objective aspect of the Tree of Life manifesting the seven Sephiroth of Construction. Of the six triangles, only one triangle is completely above this line. It corresponds to the single chord of four notes. The complete correspondence between the ten octaves and the Tree of Life is summarised below:
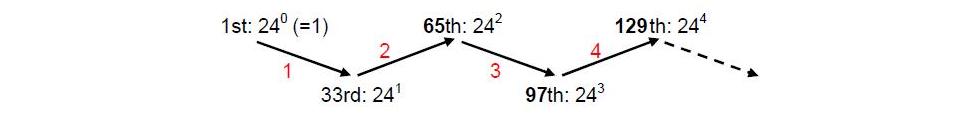
Any set of ten successive octaves exhibits this same Tree of Life pattern because the tone ratios of corresponding notes in successive octaves differ by a factor of 2, which means that, relative to the first note of any such set, there are always ten overtones with the same set of values as that found above for the first ten octaves, starting with a tone ratio of 1 for the tonic of the first octave. The perfect fifth of the fifth octave, counting from any given octave, is still an overtone with the tone ratio of 24 relative to the tonic of that starting octave. The numbers in the table of tone ratios are not absolute pitches but frequencies defined relative to that of the fundamental, which is normally given the convenient value of 1. Their underlying Tree of Life pattern is, therefore, not dependent 12 on a particular starting point but holds for any set of ten successive octaves. The fact that most of their notes would fall outside the audible range of the human ear is irrelevant. Counting from the tonic of the first octave, the tone ratio 24 (=1×2×3×4) is the 33rd note (33=1!+2!+3!+4!) and the perfect 5th of the fifth octave. Counting from the latter, the 33rd note is 576 = 242 and still the perfect 5th of the new fifth octave. This is the 65th note from the tonic of the first octave, where 65 is the 33rd odd integer. The Godname Adonai with number value 65 prescribes sequences of 33 notes whose last note has a tone ratio always 24 times that of the first note. Only the first sequence (1) has ten overtones.
The second sequence (2) has 24 overtones in addition to the first overtone with tone ratio of 24. In general, the nth sequence terminates in the note with tone ratio 24n. Notice that the Godname of Netzach, the fourth Sephirah of Construction with number value 129, determines the end of the fourth sequence with tone ratio 244, that is, (1×2×3×4) raised to the fourth power. This shows the principle of the Pythagorean Tetrad at work. The 1680 coils of each whorl in the UPA superstring have been shown in previous articles to be due to 24 gauge charges of the superstring gauge symmetry group E8, the total number of 240 for all ten whorls corresponding to its 240 non-zero roots. The number of yods in the lowest n Trees of Life is given by Y(n) = 50n + 30. The lowest 33 trees have Y(33) = 1680 yods. This is the same number as 24 separate Trees of Life, each with 70 yods, because 1680 = 24×70. Just as the first 33 notes culminate with the tone ratio 24, so the first 33 overlapping Trees of Life have as many yods as 24 separate trees. This demonstrates the association of the numbers 33 and 24 in the context of the Tree of Life. Ten objects:
arranged in a tetractys can be arranged in 13 their separate rows in 33 ways (see above). 24 of these are permutations of the last row of four objects. In this case, the 33rd permutation is the last of these 24 arrangements.
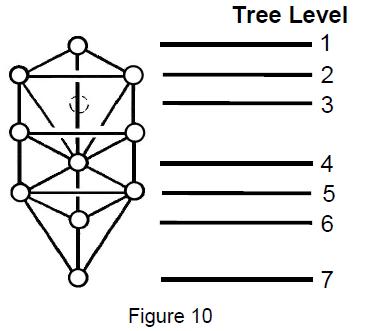
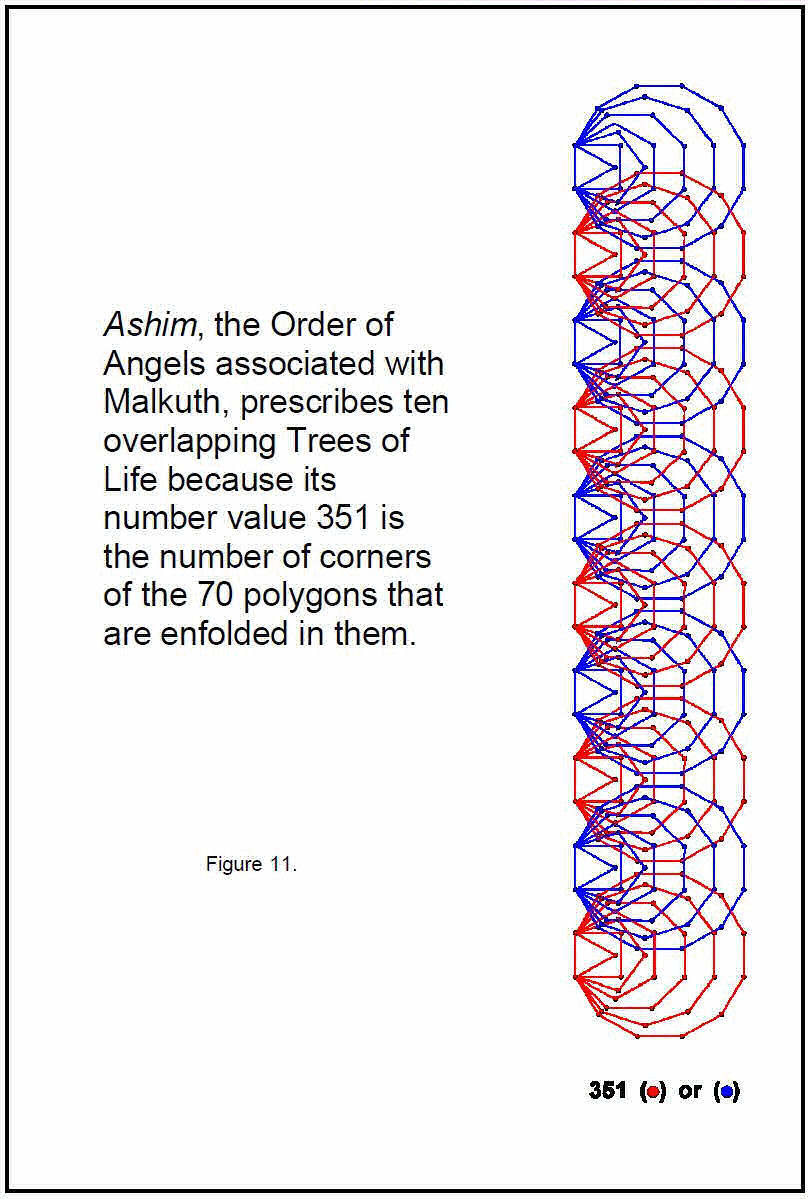
Confirmation that the number 33 represents a cycle of completion of a Tree of Life pattern of which the ten overtones spanning 33 notes is an example comes from the concept of tree levels. The emanation of the ten Sephiroth takes place in seven stages (Fig. 10). Each Sephirah can be represented by a Tree of Life. Ten overlapping Trees of Life have 33 tree levels. This number thus parameterizes the complete emanation of ten trees. Nine tree levels extend down to the top of the seventh tree, marking the last of the 25 dimensions of space. Below them are a further 24 tree levels representing the 24 spatial dimensions at right angles to the direction in which the 1-dimensional string extends. This 9:24 differentiation in tree levels separating the purely physical plane from superphysical subplanes corresponds to the nine permutations of 1, 2 and 3 objects in the first three rows of a tetractys and the 24 permutations of the four objects in the fourth row. This is in keeping with the four rows of the tetractys symbolising the four fundamental levels of Divine Spirit, soul, psyche and body, i.e., the last 24 tree levels of the 33 tree levels determine the physical form of a superstring because they represent geometrical degrees of freedom as the dimensions or directions of space along which its whorls can vibrate. Further confirmation of the cyclic nature of the number 33 in defining repeated Tree of Life patterns is that there are 33 corners outside the root edge of every successive set of
14 15 16 seven polygons enfolded in overlapping Trees of Life (Fig. 11). In general, the number of corners of the 7n polygons enfolded in n trees = 35n + 1, ‘1’ denoting the highest corner of the hexagon enfolded in the nth tree (the highest and lowest corners of each hexagon are shared with its adjacent hexagons). Hence, the 70 polygons enfolded in 10 trees have 351 corners (Fig. 11). This the number value of Ashim, the Order of Angels of Malkuth. As a set of (7+7) polygons has 70 corners, there are 64 corners outside their root edge that are unshared with hexagons in adjacent sets. This is the number of Nogah, the Mundane Chakra of Netzach, the Sephirah energising the music and art of the soul. Another remarkable property of the number 33 is that the 33rd prime number is 137. This is one of the most important numbers in theoretical physics (3) because its reciprocal is almost equal to the so-called “fine-structure constant.” This determines the probability that an electron will emit or absorb a photon and measures the relative strength of the electromagnetic force compared with the nuclear force binding protons and neutrons together inside atomic nuclei. As yet theoretically undetermined by physicists, the number 137 is encoded in the inner form of the Tree of Life as the 137 tetractyses whose yod population is equal to the yod population of the (7+7) enfolded polygons when their sectors are each transformed into three tetractyses (Fig. 12). 1370 is also the number of yods in 27 overlapping Trees of Life (4), which gives another remarkable significance to the largest number in Plato’s Lambda, which is prescribed by the Godname Yahweh as the 26th number after 1. Indeed, 27 can be said to define the ten octaves of the Pythagorean scale in the
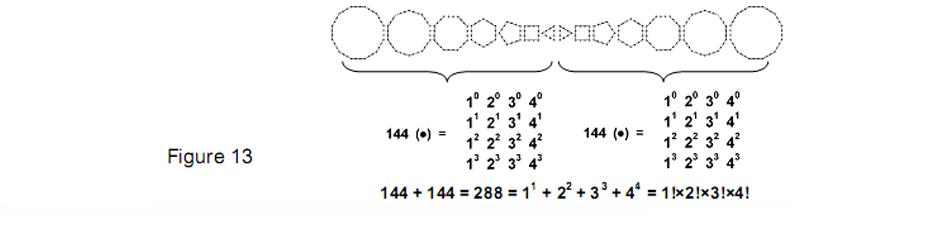
sense that 972, the largest of their overtones, is the 27th overtone following the overtone 27, which is the 33rd note after the fundamental. Remarkably, 972 is also the 33rd even overtone. A tetractys of ten objects has (1!+2!+3!+4!=33) permutations of the objects in its rows. A tetractys of ten different notes would generate one note and 32 melodic intervals and broken chords formed from the other three rows of notes (see p. 22 for the definition of these musical terms). Suppose that we were to play one note, next a melodic interval, 17 THE 36 CORNERS OF THE 7 ENFOLDED POLYGONS DENOTE THE 36 Figure 14 18 19 then a broken chord of three notes and finally a broken chord of four notes. The number of possible ways of playing ten notes in succession by following the pattern of the tetractys is 1!×2!×3!×4! = 288.* This is the number of yods lying on the boundaries of the (7+7) regular polygons constituting the inner form of the Tree of Life (Fig. 13). Supposing that the notes are arranged in the tetractys in either ascending or descending order, there are 144 arrangements of ascending notes and 144 arrangements of descending notes. They have their parallel in the two similar sets of seven polygons that are shaped by 144 yods on their 48 sides. Now suppose that we were to play one note, then either a harmonic or a melodic interval, next either a chord or a broken chord of three notes and finally a chord or broken chord of four notes. The numbers of possible musical sequences created by playing 1, 2, 3 & 4 notes would be:
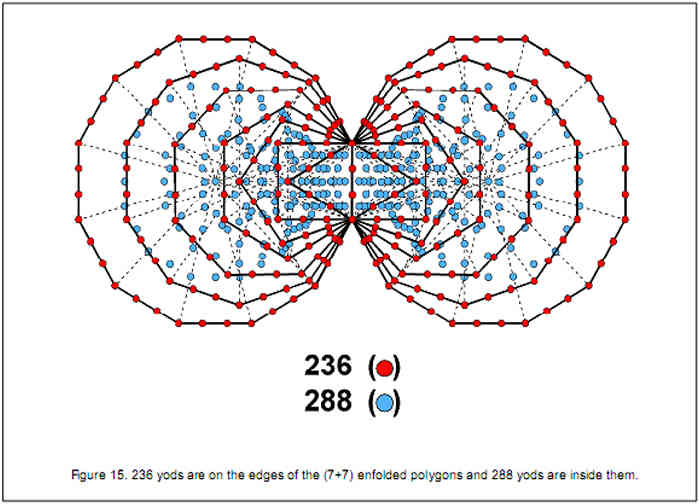
The total number of musical elements is 36, which is the number value of Eloha, the Godname of Geburah (Fig. 14). Playing 10 notes in the order of the rows 1, 2, 3 & 4 would generate (1×3×7×25=525) possible sequences of notes. One of them is the sequence of one note, one harmonic interval and two chords, i.e., four musical sounds. This is the minimum number of sounds created by playing the tetractys of 10 notes, leaving 524 sequences, each with 5-10 sounds drawn from the 36 musical elements. Compare this with the facts that the seven enfolded polygons in the inner form of the Tree of Life have 36 defining corners and that the (7+7) enfolded polygons have 524 yods. Moreover, there are (1!×2!×3!×4!=288) sequences of 10 successive sounds consisting of a note, one melodic interval and two broken chords, leaving (524–288=236) sequences with 5-9 sounds. These correspond to the 288 yods inside the (7+7) polygons and to the 236 yods on their sides (Fig. 15)! We see that the Tree of Life blueprint is inherent in the musical potential of 10 notes played in four steps according to the pattern of a tetractys, the symbol of the ten-fold nature of God (6). 3. The seven notes of the Pythagorean scale The first seven notes of the musical octave:
*288 = 172 – 1 = 3 + 5 + 7 + … + 33, where 33 is the 16th (16=42) odd integer after 1. 20 have (27–1=127) different combinations, where 127 is the 31st prime number. This indicates how the Godname El with number value 31, which is assigned to Chesed, the first Sephirah of Construction, prescribes how many groups of notes can be played together to make basic musical sounds using the 7-fold musical scale. These combinations comprise the seven notes themselves and (127–7=120) harmonic intervals and chords, where 7 is the fourth odd integer (also the fourth prime number) and 120 = 22 + 42 + 62 + 82. This illustrates how the Pythagorean Tetrad, 4, determines both the numbers of notes and their combinations. The number of harmonic intervals is 7C2 = 21, which is the number value of the Godname Ehyeh assigned to Kether. The number of chords is therefore (120–21=99). This distinction between intervals and chords is arithmetically defined as follows:
In other words, the Pythagorean character of the number 120 is shown by its being the sum of the first ten odd integers after 1, 21 being the tenth odd integer after 1 and 99 being the sum of the remaining 9 integers in this tetractys array. 99 is the 50th odd integer, showing how the Godname Elohim with number value 50 defines the number of chords that can be played with the first seven notes of the Pythagorean scale. Successive octaves comprise seven notes per octave and the eighth note beginning the next octave. N octaves therefore span (7N+1) notes. The number of ‘Sephirothic levels’ (SLs) in the lowest, n overlapping Trees of Life is (6n+5). For what values of N and n are the number of notes and SLs the same? The only solutions to: 7N + 1 = 6n + 5 up to N =10 can be found by inspection to be N = n = 4 or N = 10 & n = 11, i.e., four octaves have as many notes (29) as the lowest four Trees of Life, whilst ten octaves have as many notes (71) as eleven such Trees of Life have SLs. Every eighth note in successive octaves is of the same type, whilst every seventh SL in successive Trees corresponds to the same Sephirah. The Pythagorean Tetrad and Decad define analogous successions of notes of the scale and the emanations of Sephiroth in overlapping Trees of Life. Excluding the highest note belonging to the next higher octave, four and ten octaves have, respectively, 28 and 70 notes, the same as the SLs in four and eleven overlapping Trees of Life. In general, the counterpart of the last note of the 21 Nth octave shared with the next higher octave is Daath of the nth tree, which is Yesod of the (n+1)th tree but which is not counted as an SL when the overlapping trees are considered as a separate set. 4. Tetractys of ten notes A harmonic interval is two notes played together. A melodic interval is two notes played one after the other. A chord is three or more notes played simultaneously and a broken chord is a set of three of more notes played in succession. The following discussion will consider only melodic intervals and broken chords where the notes are all different. Consider a tetractys array of ten different notes:
(Any notes can be considered here — the letters labelling them do not refer to the notes of the Pythagorean scale). The number of intervals and chords that notes within the same row generate when played will now be determined. A harmonic interval is a combination of two notes, whereas a melodic interval is two notes played with regard to their order in time, i.e. a permutation of two notes. A chord is a combination of three or more notes, and a broken chord is a pattern of three or more notes played in quick succession, i.e., a particular arrangement or permutation of these notes. The table below shows the numbers of harmonic intervals and chords (combinations of notes) and melodic intervals and broken chords (permutations of notes) for the notes in the four rows of the tetractys:*
The number value 26 of Yahweh, the Godname of Chokmah, is the number of notes, harmonic intervals and chords that can be played within the four rows of notes, the number value 15 of its older version, Yah, being the number that can be played from four * Notation: nCr = n!/r!(n-r)! and nPr = n!/(n-r)! 22 notes. There are (26–10=16=42) harmonic intervals and chords (10 intervals & 6 chords). The number of notes, melodic intervals and broken chords is 84 = 12 + 32 + 52 + 72.
This illustrates the defining role of the Pythagorean Tetrad because 1, 3, 5, & 7 are the first four odd integers. The number of melodic intervals and broken chords = 84 – 10 = 74, which is the 73rd integer after 1. The number value 73 of Chokmah determines the number of basic musical elements (namely, melodic intervals and broken chords) that can be played from sets of 1, 2, 3 and 4 notes. The number of harmonic and melodic intervals, chords and broken chords = 16 + 74 = 90, and the number of notes, intervals and chords of both types = 90 + 10 = 100 = 13 + 23 + 33 + 43. This shows how the Pythagorean integers 1, 2, 3, & 4 express the total number of musical sounds created by playing notes from each row of the tetractys. These results can be represented by a tetractys array of the number 10: 10 10 10 10 10 10 The central number 10 represents the ten notes and the sum 90 of the remaining 10’s is the number of intervals and chords that they can create. This beautiful result demonstrates the power of the tetractys and the role of the Tetrad in defining its properties, whatever the nature of the things symbolised by its yods.
23 The three tetractyses have (3×90=270) intervals and chords. As the same notes appear in each array, the number of notes, harmonic and melodic intervals, chords and broken chords that can be played using the three orientations of a tetractys of notes = 10 + 270 = 280. The number value 280 of Sandalphon, Archangel of Malkuth, measures how many basic musical sounds composed of up to four notes can be created from ten notes arranged in a tetractys, only the notes in a row being played.
The number of harmonic intervals in each array = 2C2 + 3C2 + 4C2 = 10. The three arrays have (3×10=30) such intervals, where 30 = 12 + 22 + 32 + 42. The number of melodic intervals in each array = 2P2 + 3P2 + 4P2 = 20. The three arrays have (3×20=60) melodic intervals. The number of notes and harmonic intervals = 10 + 30 = 40
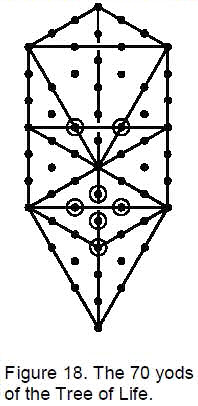
24 showing how the Tetrad determines this number, for it is the sum of the first four powers of 3. The number of notes and melodic intervals in the three arrays = 10 + 60 = 70. Compare this with Figure 18, which shows that turning the 16 triangles of the Tree of Life into tetractyses generates 60 yods in addition to those at their ten corners. The ten Sephirothic points can be assigned the notes and the 60 other yods can be assigned the melodic intervals that they generate. We have seen that the number of harmonic and melodic intervals and chords and broken chords is 270. The number of harmonic intervals, chords and broken chords = 270 – 60 = 210
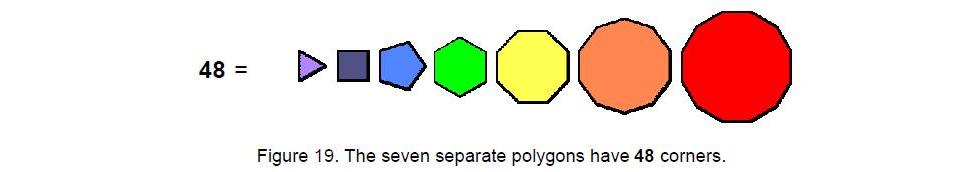
The number value 21 of Ehyeh determines how many harmonic intervals, chords and broken chords the ten notes can create. The number of chords in each array = 3C3 + 4C3 + 4C4 = 6, that is, 3×6 = 18 in the three arrays. The number of broken chords in the three arrays is therefore 210 – 18 – 30 = 162 (54 per array). The number of chords and broken chords = 18 + 162 = 180 (60 per array). The number of harmonic intervals and chords = 30 + 18 = 48 (16 per array). In other words, the number of different combinations of the ten notes (i.e., new sounds) that can be played simultaneously when selected from their three possible tetractys arrays is the same as the number of corners of the seven, separate regular polygons (Fig. 19). This illustrates the character of the number 48* (the number of Kokab, the Mundane Chakra of Hod) in quantifying the most basic
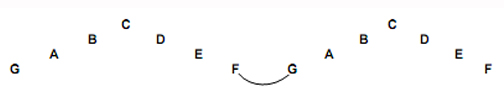
degrees of freedom making up a Tree of Life pattern — in this case, the corners of the seven polygons. The same number appears in the context of what the ancient Greeks called ‘tetrachords.’ * 48 shows its Pythagorean character by being the smallest integer with ten factors, including 1 and itself. 25 They did not experience the musical octave as one complete whole but rather as a two-part structure (5). The octave evolved through the completion of two groups of four notes, or tetrachords. For example, the sequence of notes G, A, B, C below is a tetrachord:
They shared a central note that was always a perfect fourth with respect to the beginning of the first tetrachord (G here) and the endnote of the second tetrachord (here F). The number of permutations of four objects taken one, two, three and four at a time = 4P1 + 4P2 + 4P3 + 4P4 = 4 + 12 + 24 + 24 = 64 = 43. The number 64 is the number value of Nogah, the Mundane Chakra of Netzach (astrologically associated with the planet Venus). The number of permutations of four objects taken two, three and four at a time = 64 – 4 = 60. The number of permutations of four objects taken two at a time = 4P2= 12. Hence, each of the two tetrachords in an octave has 12 melodic intervals and (60–12=48) broken chords, the latter comprising 24 (=1×2×3×4) broken chords of three notes and 24 broken chords of four notes. There are therefore two chords each of four notes and (48+48=96) possible broken chords in an octave split up into two tetrachords. Compare these divisions with the fact that the (6+6) enfolded polygons have two corners of their shared root edge and 48 corners outside their root edge, 24 on each side of it, whereas, when separated by the root edge, each set of all seven separate polygons also has 48 corners (Fig. 20). As this set of 12 polygons constitutes a Tree of Life pattern in its own right (see Article 8), we see that the ancient Greek depiction of the octave as two tetrachords conforms to the pattern of the Tree of Life. Elohim prescribes the seven polygons and root edge because, as Figure 20 shows, its number value 50 is the number of their corners (two belong to the root edge). Of the 162 broken chords generated by the three orientations of a tetractys of ten notes, 26
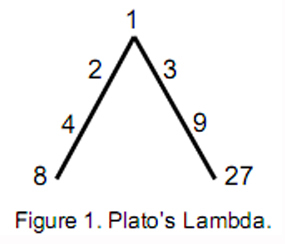
six are descending and ascending tetrachords (two per orientation). Therefore, there are (162–6=156) broken chords whose notes are not all in descending or ascending sequence. 156 is the 155th integer after 1. This is how Adonai Melekh, the complete Godname of Malkuth with number value 155, measures the number of sounds that can be made by playing the [3×(3+4) = 21] notes in the rows of three and four of the three tetractyses one after the other but not in order of their pitch. 21 is the number value of Ehyeh, Godname of Kether. 5. The Platonic Lambda revisited In Article 11, we found that the tetractys form of Plato’s Lambda:
is but one face of a tetrahedron whose fourth face is a tetractys that generates in a symmetric way the tone ratios of the Pythagorean musical scale. Properties of this parent tetractys are compared below with the various numbers of intervals and chords generated from a tetractys array of ten notes.
We find that the numbers making up the Lambda tetractys do more than define the tone ratios of musical notes — a function known to musicians and mathematicians for more than two thousand years. They also measure the various numbers of musical elements that can be played by using the four rows of different notes arranged in a tetractys. 27
28 The number 90 is 10P2, the number of permutations of two objects taken from a set of ten objects, i.e., in this context the number of melodic intervals that can be played with ten different notes without regard to their arrangement in a tetractys. In the context of the UPA superstring, a point on each of its ten whorls has (10×9=90) coordinates with respect to the 9-dimensional space of the superstring. Noting that Besant & Leadbeater states in their book Occult Chemistry (6) that none of the whorls ever touched one another as they observed them, this means that these ten, non-touching curves require 90 independent (but not necessarily all different) numbers as free coordinate variables. As discussed in Article 12, 90 is the number of trees above the lowest one in what the author calls the ‘Cosmic Tree of Life,'* i.e., the number of levels of consciousness beyond the most physical level represented by the lowest tree. This means that a musical sound containing up to four notes can be assigned to each of these levels of consciousness, with the tetractys of ten notes itself assigned to the 91st level. The counterpart of the latter for the superstring would be the time coordinate, the number that locates it in time. Alternatively, a melodic interval generated from ten notes can be assigned to these levels. As 7P2 = 42, there are 42 such intervals generated from seven notes sited at the centre and corners of the hexagon in the tetractys and (90–42=48) intervals generated by pairing either these notes with those at the corners of the tetractys or the latter themselves. This 48:42 division corresponds to the 48 subplanes of the cosmic physical plane above the lowest one and the 42 subplanes of the six superphysical cosmic planes. Whether or not this correlation may have deeper significance, it demonstrates a beautiful, mathematical harmony between the permutational properties of ten objects arranged in a tetractys and what the author has shown in previous articles to be the map of all levels of reality. The latter itself is a tetractys with the fractal-like quality that each of the nine yods surrounding its centre is a tetractys and that the central yod is the repetition of this on a spiritually lower but exactly analogous level. The five types of musical elements are notes of the Pythagorean scale, their harmonic intervals, melodic intervals, chords & broken chords. Their numbers are shown below:
(The second number in each bracket is the number of musical elements per orientation) * See Article 5 for details about this map of the spiritual cosmos. 29 There are (25–1=31) combinations of these elements, where 31 is the number value of El (“God”), the Godname assigned to Chesed, the first Sephirah of Construction. Some of these were discussed above. In order of increasing size, these numbers are: 10, 18, 28, 30, 40, 48, 58, 60, 70, 78, 88, 90, 100, 108, 162, 172, 180, 190, 192, 202, 210, 220, 222, 232, 240, 250, 252, 280. Notice that the 21st number is
and that the 28th (last) number is
The 26th number is 250. Hence, the Godname Yahweh with number value 26 marks out the number of notes, melodic intervals, chords and broken chords. This significance of this is as follows: the number 250 comprises 10 notes, 60 melodic intervals and 180 chords and broken chords. This number was found in Article 11 to be the sum of the 16 (=42) tone numbers making up the four faces of the tetrahedron other than its corners. The significance of 250 for superstrings was discussed in this and earlier articles. * Its relevance to the Tree of Life is that the 60 polygons of the first six types enfolded in ten overlapping Trees of Life have 250 corners unshared with polygons enfolded in the next higher tree (Fig. 21). They comprise the 10 lowest and highest corners of the ten hexagons, the 60 corners of the triangle, square and pentagon that are outside their root edges and 180 corners of the hexagon, octagon and decagon. The (6+6) polygons constitute a Tree of Life pattern because they have 50 corners prescribed by the Godname Elohim with number value 50 (Fig. 20; see Article 4 for how the other Godnames prescribe their geometry). It is a remarkable demonstration of how Godnames are fundamentally connected to the tetractys that the number of Yahweh should pick out from all possible combinations of basic musical elements (themselves numbered by the Godname of the Sephirah lying below Chokmah) just that number which is also the number of geometrical degrees of freedom denoted by corners of polygons enfolded in * In particular, see Article 5. 30 ten Trees of Life! Not five trees, nor nine trees, but precisely the Pythagorean and Kabbalistic measure of Divine Perfection, namely, the number 10! The number of melodic intervals, chords and broken chords = 250 – 10 = 240
Suppose that we consider a tetractys of nine notes with the central one missing:
The numbers of musical elements now become:
(The second number in each bracket is the number of elements per orientation) The three tetractys arrays possess 240 notes, harmonic intervals,
melodic intervals, chords and broken chords — the same number as the number of melodic
intervals, chords and broken chords generated by a complete
tetractys of ten notes. In terms of the correspondence between the tetractys
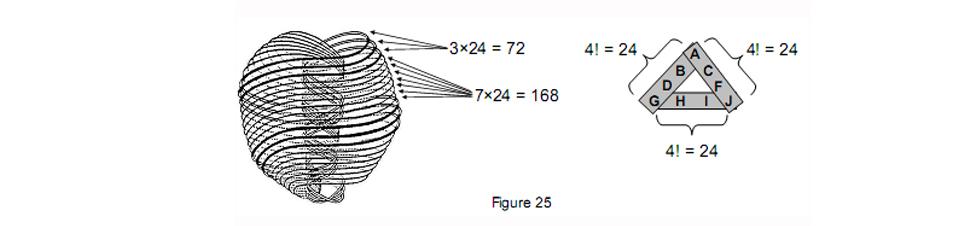
and the Tree of Life, the central yod symbolises 31 Malkuth, the outer, physical realisation of a Tree of Life entity (superstrings, human beings, etc). What the reappearance of the number 240 in a tetractys of notes with the central one missing is saying is that 240 of the original 280 musical elements are due to those notes that formally correspond to Sephiroth above Malkuth. This accounts for why the Archangel of Malkuth has the number value 280. 6. The musical nature of superstrings The 240 musical elements generated from the nine notes corresponding to Sephiroth above Malkuth comprise (24+48=72) intervals* and (9+15+144=168) notes, chords and melodic chords. This division is reflected in the above tetractys representation of the number 240 because its three corners sum to 72 and the seven remaining numbers 4! add up to 168. Earlier articles and the author’s book (7) have established that each of the ten whorls of the UPA superstring (Fig. 22), observed with a yogic siddhi called ‘anima’ by Annie Besant and C.W. Leadbeater, carries 24 gauge charges of the unified, superstring gauge symmetry group E8 corresponding to the latter’s non-zero simple roots. The three ‘major’ whorls carry (3×24=72) such charges and the seven ‘minor’ whorls carry (7×24=168) charges. The former are in fact the charges corresponding to the 72 non-zero * The alternative combination of 72 notes, harmonic intervals and chords is less plausible, intuitively speaking, because it mixes musical elements of different types. 32
simple roots of E6, an exceptional subgroup of E8, there being 168 non-zero roots of E8 that do not belong to E6. Remarkably, the musical elements due to the notes corresponding to Sephiroth above Malkuth correspond precisely in number to the gauge charges mathematically associated with the non-zero roots of E8 and its subgroup E6. Transformed into single tetractyses, the lowest Tree of Life contains 80 yods (Fig. 23). Using the numbers (shown above in brackets) of musical elements of each type generated by a tetractys of ten notes, the total number of such musical elements is (3 + 8 + 16 + 5) + 48 = 32 + 48 = 80. Each yod in the lowest Tree of Life can denote a musical element! Moreover, there are 48 yods up to the level of Chesed, the first Sephirah of Construction, illustrating once again the nature of this number in quantifying the number of formative degrees of freedom that are needed to express the part of the Tree of Life that manifests in an objective sense. The 48 yods up to Chesed denote the number of broken chords and the 32 yods above this level denote the number of notes, intervals and chords. In this remarkable way, the number of musical sounds (80) created by the notes corresponding to the nine Sephiroth above the objective one, Malkuth, and their division into melodic chords of three and four notes (the beginning of melody) reflects precisely the 48:32 pattern of the lowest Tree. The number 240 is the number of yods generated in the lowest Tree of Life when its 19 triangles are
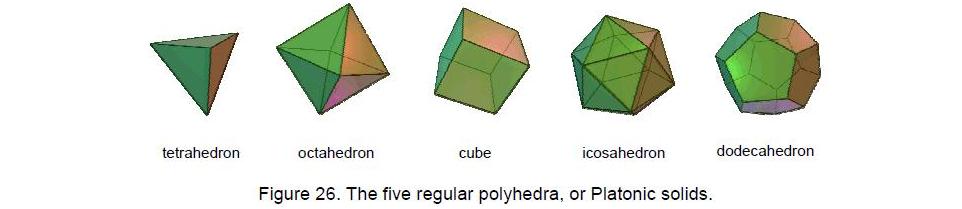
transformed into three tetractyses, that is, yods in addition to its 11 Sephirothic points (Fig. 24). These 240 hidden or potential degrees of freedom correspond to the potential of 240 musical elements that can be played with the set of nine notes corresponding to the Sephiroth above Malkuth. Notice that the correspondence is both qualitative and quantitative because the 11 Sephirothic points of 33 the lowest tree constitute its most basic outer form (its Malkuth aspect), so that the hidden 240 yods represent what is beyond this aspect, just as the 240 musical elements denote sounds generated by notes corresponding formally to Sephiroth above Malkuth. A 72:168 pattern similar to that found above for three tetractyses with their central note missing also exists when the latter is present. The number of melodic intervals, chords broken chords with three notes. The (3×80=240) melodic intervals, chords and broken chords generated by the three orientations of the tetractys comprise (3×24=72) broken chords of four notes and (3×56=168) melodic intervals, chords and broken chords of three notes. It mirrors the 72 E8 gauge charges carried by the three major whorls and the 168 such charges carried by its seven minor whorls (Fig. 25). In terms of the charge sources of all the forces other than gravity that superstrings of ordinary matter can exert on one another, superstring physics conforms to the same pattern as the melodic sounds generated by playing the ten notes of a tetractys in its three orientations. 7. The Platonic solids represent the 90 musical elements In Article 3 it was shown how, when seen as constructed from the tetractys (the Pythagorean symbol of ten-fold Divine unity), the first four regular polyhedra embody numbers of significance to the mathematics of superstring forces. It will now be proved that the five Platonic solids (Fig. 26) also embody in a geometrical way the numbers of different types of musical sounds that can be generated from a tetractys of ten notes.
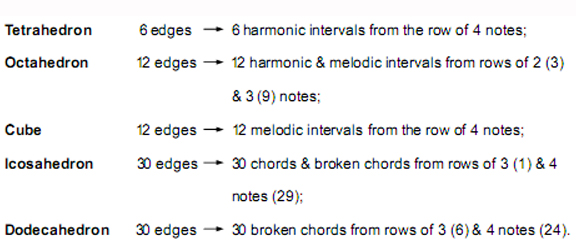
The numbers of edges in the five Platonic solids are shown below:
The total number of edges is 90, which is the same as the number of musical elements generated by a tetractys array of ten notes, that is, sounds other than the notes themselves. They comprise six harmonic intervals generated from the row of four notes, 34 12 harmonic and melodic intervals generated from the rows of two and three notes, 12 melodic intervals generated from the row of four notes, 30 chords and broken chords of three or four notes generated from the rows of three and four notes (six chords, 24 broken chords of three notes from the row of four notes) and 30 broken chords (six from the row of three notes, 24 from the row of four notes). The correspondence between the edges of the Platonic solids and these musical elements is:
(Numbers in brackets are the numbers of musical elements generated by the stated row) Notice how the complexity of the musical elements builds up correspondingly with the number of edges of the Platonic solids. They start with the harmonic intervals (the simplest musical sounds), which correspond to the edges of the tetrahedron, the simplest Platonic solid, and finish with the broken chords (the most complex sounds), which correspond to the edges of the dodecahedron, the last of the regular polyhedra. This natural progression argues against the agreement between the various numbers of edges and musical elements being merely a coincidence or a concoction. The Godname Elohim with number value 50 assigned to the Sephirah Binah prescribes both the form of the Platonic solids and the set of ten notes and their musical combinations because the former have 50 corners and the latter comprise 100 musical elements in one array, where 100 is the 50th even integer. They include 26 single sounds (notes, harmonic intervals and chords), where 26 is the number value of Yahweh, the Godname of Chokmah. The number of notes, melodic intervals and broken chords generated by a tetractys of notes is 84. As 84 = 3×28 = 3(1+2+3+4+5+6+7) = 3 + 6 + 9 + 12 + 15 + 18 + 21, this is how the number value 21 of Ehyeh, the Godname of Kether, determines the number of such musical sounds. The number of musical sounds made up of notes played in succession is 74, which is the 36th even integer after 2. This is how 35 Eloha, the Godname of Geburah with number value 36, prescribes the number of sounds of this type. The number of broken chords of four notes generated by the fourth row of a tetractys = 4! = 24. The three possible tetractys arrays therefore have (3×24=72) such chords, where 72 is the number value of Chesed, the fourth Sephirah from the top of the Tree of Life. 72 is the 36th even integer. It is therefore also prescribed by Eloha. 8. The octave as analogue of the rank-8 gauge group E8 The number of combinations of the eight notes of the
musical scale = 28 – 1 = 255 = 8C1 +
8C2 + 8C3 +
8C4 + 8C5 +
8C6 + 8C7 +
8C8. 255 = 8 + 28 + 56 + 70 + 56 + 28 + (17 + 77) + 18. 255 – 77 = 248 = 8 + 28 + 56 + 70 + 56 + 28 + 17 + 18 ‘8’ denotes the first seven notes and the octave. 248 – 8 = 240 = 28 + 56 + 70 + 56 + 28 + 17 + 18. = (56 + 56) + 28 + 70 + 28 + 17 + 18
where 112 = 56 + 56 and 128 = 28 + 70 +28 + 17 + 18. 112 is the number value of Beni Elohim, the Order of Angels assigned to the Sephirah Hod (see ref. 1). Compare this with the fact that the superstring gauge symmetry group E8 is defined mathematically by its 248 simple roots, which comprise eight zero roots (seven of one kind and one of another kind) and 240 non-zero roots. The latter comprise 112 non-zero roots of one kind and 128 non-zero roots of another kind. We see that the following correspondence emerges between the Pythagorean scale and the root structure of E8:
*It is unnecessary to explain here their difference in the technical terms of group theory. 36
The number 240 can be also written as:
where
and
72 (the number of Chesed, the first Sephirah of
Construction) is the sum of the number (70) of chords of four notes created from the
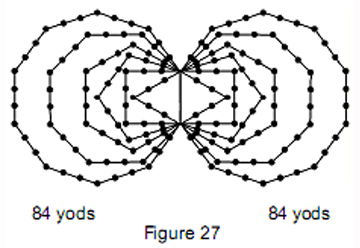
168 is the number value of Cholem Yesodoth, the Mundane Chakra of Malkuth. The 84:84 division of the number 168 that emerges naturally above from the rearrangement of combinatorial numbers is reflected in its encoding in the first (6+6) enfolded, regular
polygons of the inner form of the Tree of Life (Fig. 27) because there are 84 yods in each set of polygons outside their shared root edge*. This splitting into equal numbers is reflected in the UPA itself as the spiralling of each whorl with 1680 coils 2½ times around the axis of the UPA, returning to its top by making 2½ narrower twists (see Fig. 25). Each half of the whorl comprises 840 coils, which is the number of yods shaping the 60 polygons of the first six types enfolded on each side of the central pillar of ten overlapping Trees of Life that represent each of the ten whorls of the superstring. The division of the structural parameter 168 into two halves, as physically manifested in the inner and outer halves of the superstring, is due to the mathematical fact (referring to the equation at the beginning of this section) that 8C2 = 8C6 = 28 and 8C3 = 8C5 = 56. In terms of the octave, this means that 28 harmonic intervals and 56 chords of three notes (making 84) can be played with an octave, as can 28 chords of six notes and 56 chords of five notes (also making 84). In terms of the first six polygons, namely, the triangle, square, pentagon, hexagon, octagon and decagon, there are, respectively, 5, 8, 11, 14, 20 and 26 yods along their edges outside their root edge. The square and octagon has (8+20=28) yods and the triangle, pentagon, hexagon and dodecagon have (5+11+14+26=56) yods. For one set of polygons, the harmonic intervals can be represented by the yods of the square and octagon, whilst the chords of three notes can be represented by the yods of the triangle, pentagon, hexagon and decagon. For the other set, the square and octagon represent the chords of six notes, and the four other polygons represent the chords of five notes. No alternative combinations of polygons are possible. The musical potential of the Pythagorean octave finds its counterpart in the sacred geometry of the inner Tree of Life. 9. Conclusion The numbers of Plato’s Lambda tetractys should not be seen simply as generating the tone numbers of the Pythagorean scale and all its octaves. Their absolute, as well as their relative, values have a meaning that is both metaphysical and musical. In the former case, the central number (6), the sum (48) of the six numbers at the corners of the hexagon, the sum (84) of the numbers surrounding the centre of the tetractys and the sum (90) of all ten numbers measure, respectively, the levels of physical consciousness above the most rudimentary, the cosmic counterparts of these levels, all superphysical levels and all levels of consciousness. In the latter case, these numbers refer to the
38 various musical sounds that can be played with a tetractys of ten notes. They are, respectively, the chords, next, the broken chords of three or four notes played from the base set of four notes, then, the notes, melodic intervals and broken chords, and, finally, the harmonic and melodic intervals, chords and broken chords. The numbers of the Lambda refer to higher levels of consciousness (analogous levels in the case of the above combinations) that bear a correspondence to the basic music sounds playable with a tetractys of notes. This is the real meaning of the music of the World Soul. The number value 280 of the Hebrew name of the Archangel of Malkuth is the number of musical sounds that can be played with a tetractys of notes in its three possible orientations. The Pythagorean scale conforms to the pattern of the Tree of Life, which maps each of the 91 levels of consciousness. As the perfect fifth of the fifth octave, the tenth overtone and the 22 fractional notes that precede it complete a Tree of Life pattern. Its tone ratio 24 is central to the structure and dynamics of superstrings. Music, the study of number in time, and geometry, the study of number in space, come together in the five Platonic solids. Their 90 edges correlate with the 90 harmonic and melodic elements, chords and broken chords that can be played with a tetractys of ten notes. This number also characterises the superstring because each of its ten string components extends in nine dimensions, their oscillations described by 90 independent variables. Music, the vibrations of superstrings and the spectrum of consciousness are interrelated in universal correspondence and harmonious proportion through the Pythagorean symbol of the tenfold nature of Divine Unity.
Antoine Fabre D’Olivet, 18th century Pythagorean. 39 References
40
Only the chords DFA and EGB listed in (6) made up of alternate notes and spanning the interval of a fifth exhibit the same proportions of their tone ratios, their corresponding notes being each separated by a whole tone. Musical harmony between a pair of chords exists in this sense only for one of the ten pairs. All seven notes form 7C3= 35 chords of three notes, that is, (35–20=15) more chords than the other six notes. The number value 15 of the Godname Yah is therefore the number of chords of three notes containing the tonic. The chord EGB is the chord DFA lifted by a whole tone. Just as the six Sephiroth of Construction above Malkuth consist of two triads, so the six notes above the tonic, which is assigned to the central yod symbolising Malkuth, form uniquely two harmonious chords. The pairs of notes (E, D), (G, F) and (B, A) have the same relative tone
interval of 9/8. Note E corresponds to note D, G corresponds to F and B corresponds to A. This is consistent with their assignment:
because Netzach, which is below Chesed on the Pillar of Mercy, is its 41 counterpart in a new cycle of differentiation, whilst Hod similarly is the counterpart of Geburah below it on the Pillar of Judgement and Yesod, the psyche, is the human reflection of the spiritual individuality represented by Tiphareth lying above it on the Pillar of Equilibrium.
42 |


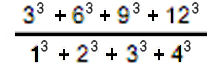





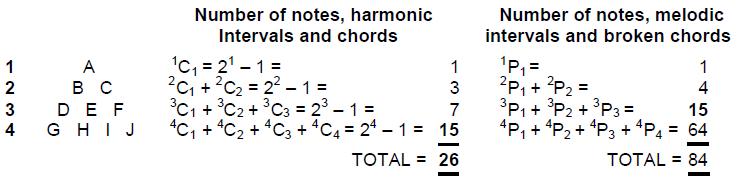





 eight notes of the Pythagorean scale, the chord of the first seven notes
(17) and the chord of all eight notes (18). 168 is the
number of harmonic intervals and chords of three, five and six notes. The point of this
exercise is that the composition of musical sounds created from the eight notes of the
Pythagorean scale can be viewed as analogous to the 72:168 division of
the non-zero roots of the superstring symmetry group E8 and their
corresponding gauge charges, which previous articles by the author have shown to be
encoded in the inner form of the Tree of Life. As discussed earlier, this difference
corresponds to the distinction between the three major whorls and the seven minor whorls
of the UPA superstring, that is, to the distinction in the Tree of Life between the
Supernal Triad (the triple Godhead) and the seven Sephiroth of Construction.
eight notes of the Pythagorean scale, the chord of the first seven notes
(17) and the chord of all eight notes (18). 168 is the
number of harmonic intervals and chords of three, five and six notes. The point of this
exercise is that the composition of musical sounds created from the eight notes of the
Pythagorean scale can be viewed as analogous to the 72:168 division of
the non-zero roots of the superstring symmetry group E8 and their
corresponding gauge charges, which previous articles by the author have shown to be
encoded in the inner form of the Tree of Life. As discussed earlier, this difference
corresponds to the distinction between the three major whorls and the seven minor whorls
of the UPA superstring, that is, to the distinction in the Tree of Life between the
Supernal Triad (the triple Godhead) and the seven Sephiroth of Construction.