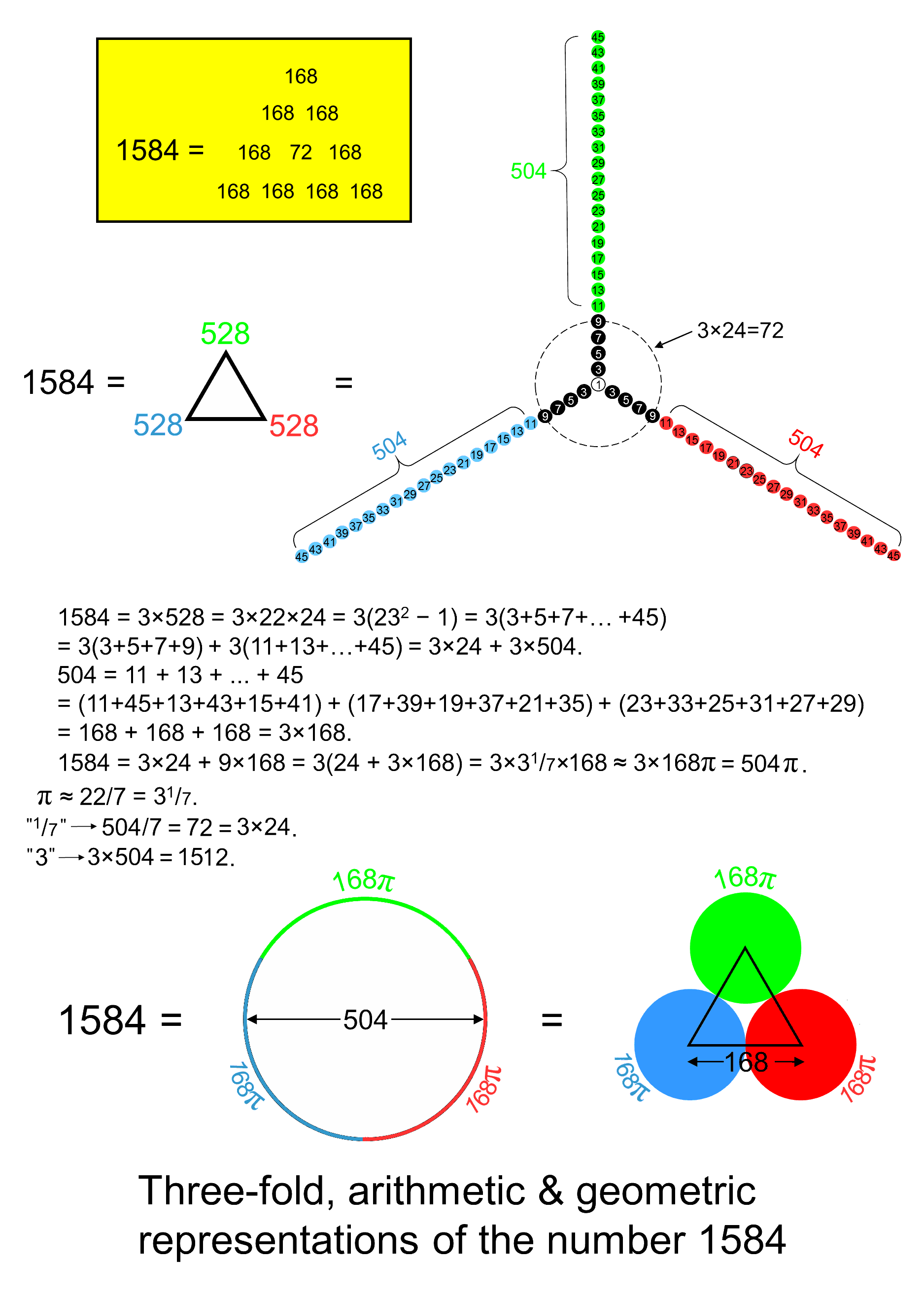
#16 Representations of the number 1584 as 3×528

We saw in #15 that the yod population of the separate outer & inner Trees of Life is 1584 and that it consists of three sets of 528 yods, each set consisting of a subset of 24 yods and a subset of 504 yods. The 3×528 factorisation of this number and the numbers 24 & 504 are determined not only by the geometries of the outer & inner Trees but also by simple arithmetic, as now shown.

As 1584 = 3×528, where

528 = 23² − 1 = 3 + 5 + 7 +... + 45

is the sum of the first 22 odd integers after 1, and

24 = 5² − 1 = 3 + 5 + 7 + 9

is the sum of the first four odd integers after 1, 528 = 24 + 504, where

504 = 11 + 13 + 15 +.... + 45

is the sum of 18 odd integers. Adding the first and last numbers, the second and next-to-last numbers, etc, we see that 504 is the sum of nine 56s that form three sets of three, each of whose sum is 168:

504 = (11+45) + (13+43) + ... + (27+29) = (56+56+56) + (56+56+56) + (56+56+56) = 168 + 168 + 168 = 3×168.

Therefore, 1584 = 3×528 = 3(24+504) = 72 + 3×3×168. The number 1584 can be represented by a tetractys with the number value 72 of Chesed at its centre and the number value 168 of Cholem Yesodoth lining its sides:

It can be also represented by a triangular array of 66 odd integers, each arm of the array containing the 22 odd integers 3-45. The sum of the first four odd integers in each arm is 24, and this corresponds to the three sets of 24 yods that make up part of the 1584 yods in the separate outer & inner Trees of Life (see #15). The sum of the 18 remaining odd integers in each arm is 504, and this corresponds to the three sets of 504 yods.

Next, consider three circles of the same radius with their centres located at the corners of an equilateral triangle. Suppose that the circles touch one another and that the length of each side of the triangle is 168 units (it does not matter what these units are). As the diameter of each circle is 168 units, its circumference is 168π units, where π = 3.14159… . People in the ancient world knew of pi but worked with various approximations of its exact magnitude. The great, ancient Greek mathematician Archimedes (c. 287 – c. 212 BCE) calculated upper and lower bounds for pi. He found that its upper bound was 22/7. This may have led to the widespread belief that pi was equal to 22/7 (= 3¹/7). This is almost true, for this number is within 0.2% of its precise value. Working with this approximate value, the circumference of each circle ≈ 168×(22/7) = 168×(3+¹/7) = 504 + 24 = 528. The sum of the circumferences of the three circles = 3×168π = 504π ≈ 504(3+¹/7) = 3×504 + 72 = 1584. We see that the well-known approximation to pi allows a natural representation of the number 1584 in terms of the 1584 units of distance approximately making up the circumferences of three circles of diameter 168. Compare it with the exact value 1583.36… of 504π. The difference is only about 0.04%, or one in 2500! Alternatively, a single circle of diameter 504 units is approximately 1584 units in circumference and can be divided into three arcs of the same length 168π ≈ 528. The fraction of "1/7 " in "3¹/7" corresponds to the number 24, and the "3" corresponds to the number 504.

The meaning of the number 504 vis-à-vis CTOL is that it is the number of SLs from its apex to the top of the 7-tree. They span all the 84 superphysical subplanes of being, there being 168 SLs on each side pillar above the 7-tree and 168 SLs on the central Pillar of Equilibrium down to (and including) its apex. A circle whose diameter is 504 units long has a circumference that is 1584 units long when the customary value 22/7 of pi is assumed. 1584 yods are needed to construct the lowest 31 Trees of Life out of tetractyses, including the four yods that lie outside them up to their apex, where 31 is the number value of EL, the Godname of Chesed. As calculated in the picture shown below, the 31st SL from the base of CTOL is the 248th yod from this point and the 137th yod from the apex of the 7th Tree. This shows how the fine-structure number 137 marks a section of the 7-tree that embodies the number of symmetries of all the forces acting between E₈′-singlet states of E₈×E₈′ heterotic superstrings. Their sum is the sum of the squares of the first 10 integers:

137 + 248 = 385 = 1² + 2² + 3² + 4² + 5² + 6² + 7² + 8² + 9² + 10².

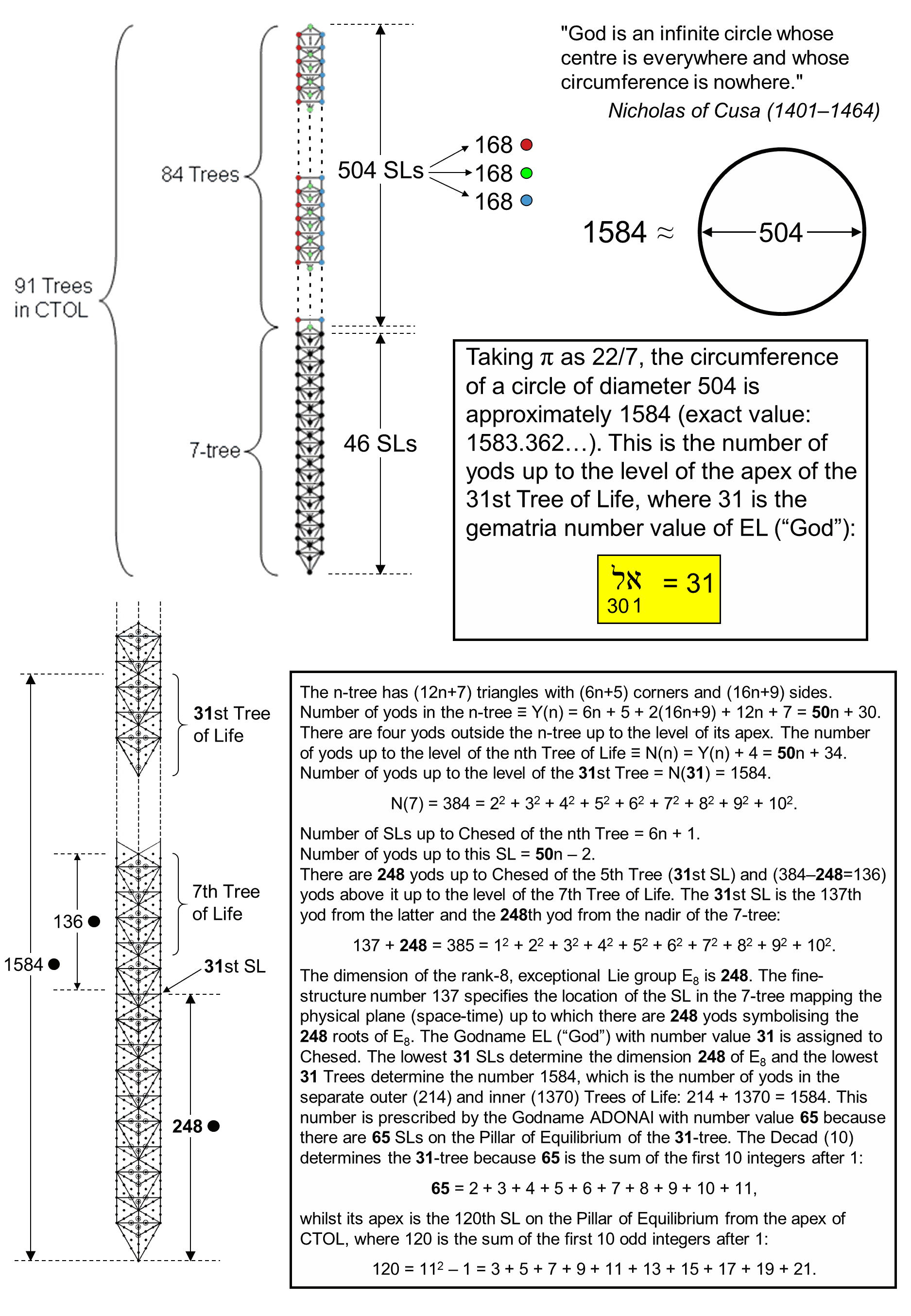
Unless one is willing to believe in miracles of chance, this beautiful property is, of course, not due to coincidence but arises from the very nature of the numbers 137 and 248 as defining parameters of sacred geometries. After all, it is because the Tree of Life is an example of sacred geometry that the 7-tree displays such arithmetic properties. Such mathematical beauty involving numbers that determine the physics of the universe to be expected in this section of CTOL that maps space-time. The sum:

136 + 248 = 384

appears in the 384 yods that belong to the first (6+6) enfolded Type A polygons in the inner form of successive Trees of Life:

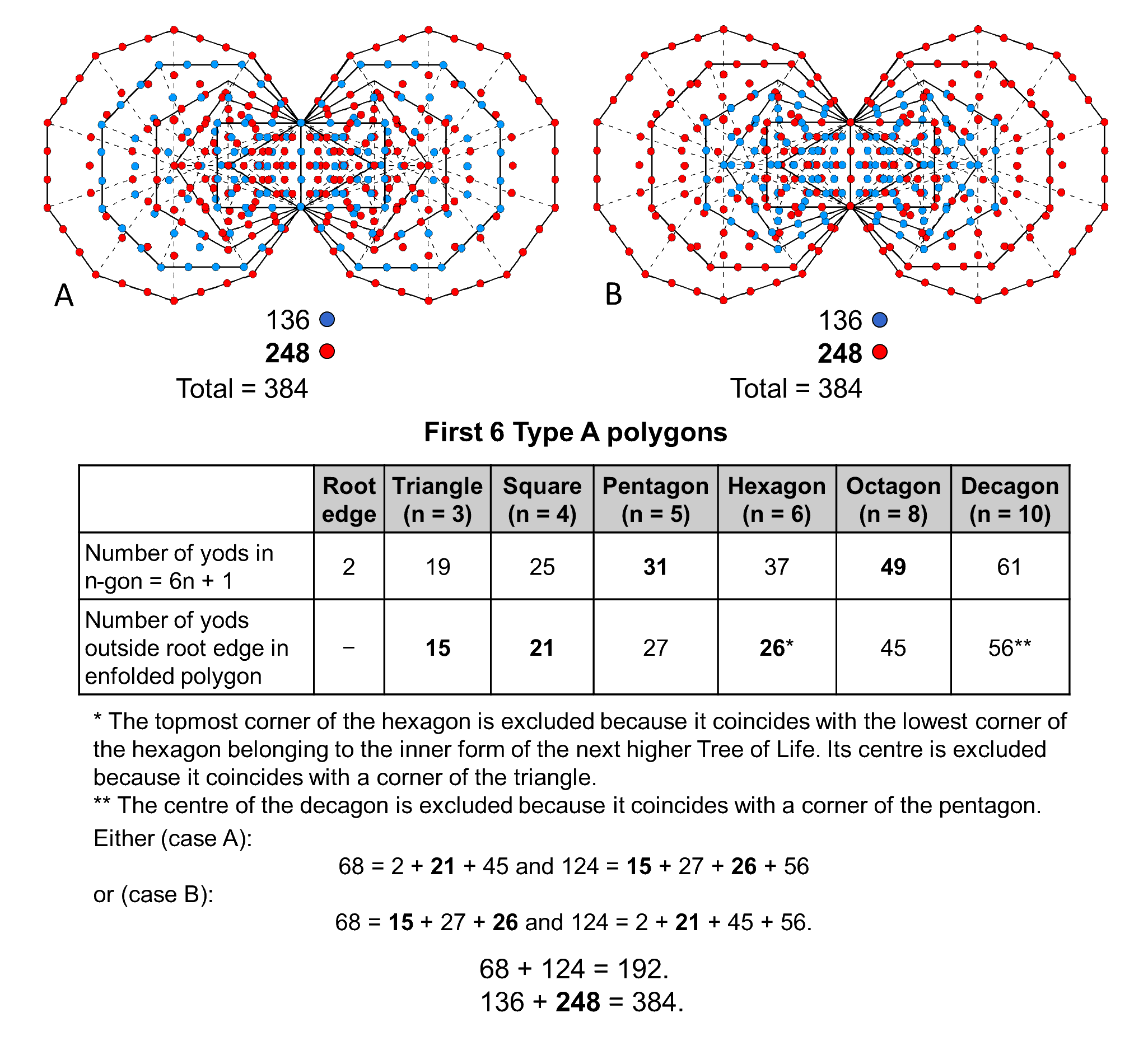
There are two possible cases:
Case A
there are 136 blue yods in the two squares & two octagons (including the root edge) and 248 red yods in the two triangles, pentagons, hexagons & decagons;
Case B
there are 136 blue yods in the two triangles, two pentagons & two hexagons and 248 red yods in the two squares, two octagons & two decagons (including the root edge).
The issue of which is the correct combination need not detain us because the point being established here that the first (6+6) polygons enfolded in successive Trees of Life contain 384 yods that can be distributed among them in such a way that they form sets of 136 and 248.

The same 136:248 division manifests in the geometrical composition of the inner Tree of Life: The (7+7) separate polygons have (192+192=384) geometrical elements surrounding their centres:

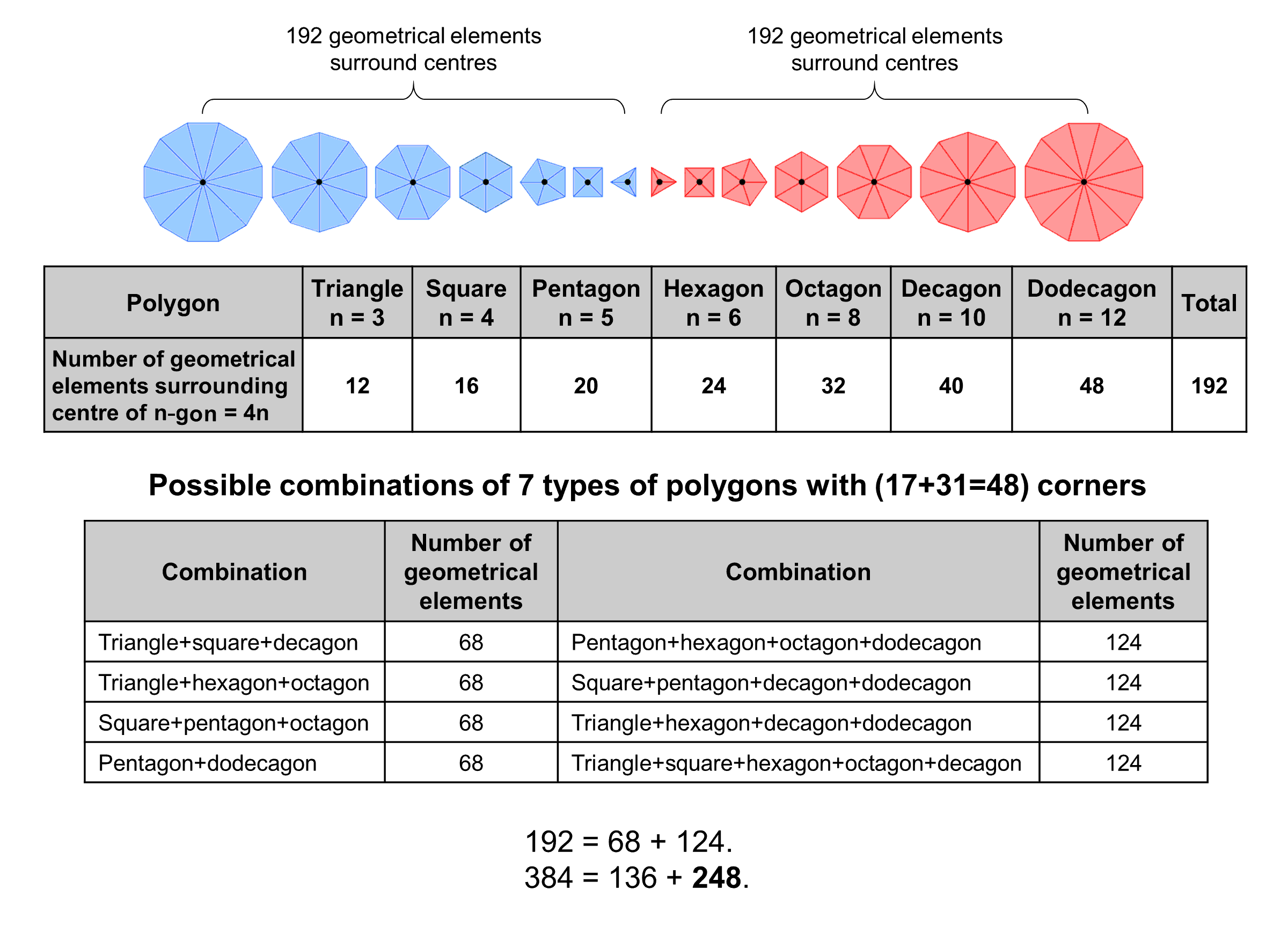
It is readily verified that there are four possible combinations of polygons that, for each set of seven separate polygons, have either 68 or 124 geometrical elements surrounding their centres. The inner Tree of Life exhibits the same 136:248 division in both its yod and geometrical composition as the 384 yods in the 7-tree do, the demarcation being prescribed by the Godname EL ("God") of Chesed.

<< Previous    1...   14  15  [16]  17  18  ...20    Next >>

Home