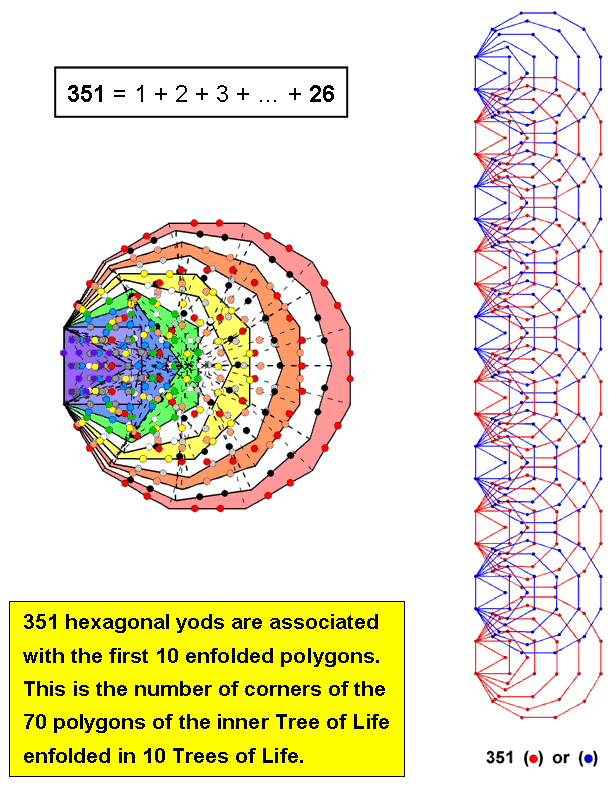
#69 The first 10 enfolded polygons embody the number of corners of the polygons enfolded in 10 Trees of Life

The seven types of enfolded polygons of the inner Tree of Life are:

triangle, square, pentagon, hexagon, octagon, decagon & dodecagon.

The dodecagon is the tenth type of polygon, counting from the triangle. The three polygons absent from the inner Tree of Life:

heptagon, nonagon & undecagon

were shown in #26 at Miscellaneous wonders to display properties that are quantified by holistic parameters found in the set of seven polygons. This suggests that, far from being merely absent from the set of polygons that form the inner Tree of Life, these three polygons have an archetypal significance as well. The 3:7 division of polygons suggests that the first 10 polygons bear a formal correspondence to the 10 Sephiroth, the three absent polygons corresponding to the Supernal Triad of Kether, Chokmah & Binah, which are the source of all levels of reality, and the seven polygons making up the inner Tree corresponding to the seven Sephiroth of Construction, which express these realities. Further evidence in support of the archetypal status of the 10 polygons is now presented.

When their 74 sectors are tetractyses, the first 10 enfolded polygons have 352 hexagonal yods (see comment 1 on the third table in #26 at Miscellaneous wonders. Two of them lie on their shared root edge. One hexagonal yod can be associated with one set of 10 enfolded polygons and the other hexagonal yod can be associated with its mirror-image counterpart. This means that 351 hexagonal yods can be associated with either set of enfolded polygons, where 351 is the number value of Ashim, the Order of Angels assigned to Malkuth (in the diagram shown opposite, the yods in the heptagon, nonagon & undecagon are coloured, respectively, white, grey & black; other yods take the colour of the polygon containing them). This number is also the 26th triangular number:

1 + 2 + 3 + ... + 26 = 351,

showing how YAHWEH, the Godname of Chokmah with number value 26, prescribes the hexagonal yod population of the first 10 enfolded polygons. One of them lies on the root edge and 350 hexagonal yods are outside it.

The seven enfolded polygons of the inner Tree of Life have 36 corners, where 36 is the number value of ELOHA, the Godname of Geburah. The topmost corner of the hexagon coincides with the lowest corner of the hexagon that is part of the seven polygons enfolded in the next higher Tree of Life. Thirty-five corners are intrinsic to the seven polygons enfolded in successive Trees of Life. The number of corners of the 7n polygons enfolded in n Trees of Life = 35n + 1, where "1" denotes the topmost corner of the hexagon enfolded in the nth tree. The 70 polygons enfolded in the lowest 10 Trees have 351 corners, of which 350 corners are intrinsic to these polygons and one is shared with the hexagon enfolded in the next higher Tree.

We see that the first 10 types of enfolded polygons embody the number of corners of the inner form of 10 Trees of Life. If we had found that the number of hexagonal yods in the former was, say, the number of corners in eight overlapping Trees of Life, this would have meant that nothing concerning the archetypal status of the 10 polygons could be inferred, for there is nothing special about the number 8 vis-à-vis the Tree of Life. However, the fact that the hexagonal yod population refers to the polygons enfolded in 10 Trees of Life is highly significant in view of the basic relevance of the Decad to the Tree of Life; 10 overlapping Trees are the next level of representation of the 10 Sephiroth of a single Tree. Not only that, the author's study of many forms of sacred geometry (see here under the heading "350 = 90 + 260") has shown that they always embody the number 350 in some way — geometrically or arithmetically, as in the case of the Tetrahedral Lambda — and it is striking confirmation of the archetypal status of the first 10 enfolded polygons that they should contain 350 hexagonal yods outside their root edge. The hexagonal yod on the root edge associated with each set of 10 enfolded polygons corresponds to the topmost corner of the hexagon enfolded in the tenth Tree. This corner has a special status because, being shared with the hexagon enfolded in the eleventh Tree, it is not intrinsic to the 70 polygons enfolded in the lowest 10 Trees. Likewise, one hexagonal yod on the root edge is only associated with one set of seven enfolded polygons; it does not exclusively belong to this set because it is also shared with the other set of polygons.

The properties of the first 10 polygons are analysed here.

<< Previous    1...   67  68  [69]  70  71  ...81    Next >>

Home