| << Previous 1... 16 17 [18] 19 20 ...50 Next >> |

The number of yods below the top of the n-tree with the sectors of triangles turned into tetractyses is given by:
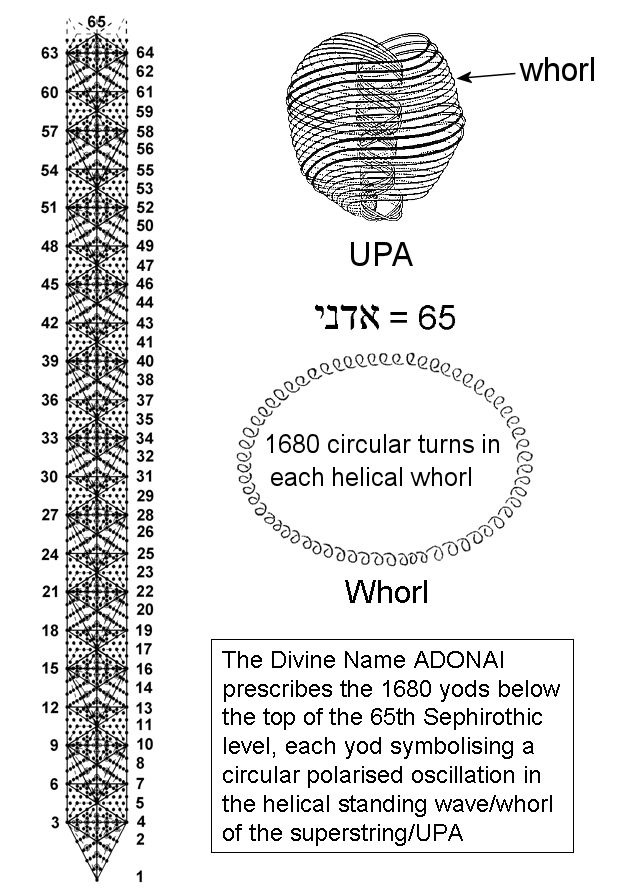
N(n) = 158n + 100.*
Hence, N(10) = 1680. In other words, 1680 yods are needed to create the lowest ten Trees below their apex, the 65th SL, where 65 is the number value of ADONAI. This is the amazing way in which this Godname of Malkuth prescribes the superstring structural parameter 1680 recorded by Charles Leadbeater when he examined the whorls of a UPA with micro-psi vision and counted their helical turns (see page 11 here). What better example could there be of how the Godnames mathematically determine those aspects of the Tree of Life that are the expression of their corresponding Sephiroth? This is one of the most remarkable examples presented in this website of how sacred geometrical objects prescribed by the Godnames embody numbers of universal significance and therefore of fundamental scientific importance. It cannot, plausibly, be coincidence that below the 65th SL are 1680 (=168×10) yods, where the numbers 65 and 168 are gematria number values referring to Malkuth, the same Sephirah!
The number of triangles in the n-tree ≡ T(n) = 12n + 7. Their number of sectors = 3T(n) = 36n + 21. The 10-tree has 127 triangles with 381 sectors, where 127 is the 31st prime number and 31 is the number value of EL, the Godname of Chesed. Of the 1680 yods below the top of the 10-tree, four yods lie outside it in two sectors on either side of the central Pillar of Equilibrium (see adjacent diagram). The 1680 yods belong to (381+2+2=385) tetractyses, where
|
12 |
||||
| 22 | 32 | |||
|
385 = |
42 |
52 |
62 |
|
| 72 | 82 | 92 | 102 . |
This demonstrates how the Decad (10) determines the amazing, beautiful properties of the 10-tree.
* Proof: the n-tree has (12n+7) triangles with (6n+5) corners and (16n+9) sides. When each triangle is a Type A triangle, it has 10 yods inside it. Number of yods in n-tree = 6n + 5 + 2×(16n+9) + 10×(12n+7) = 158n + 93. Below the apex of the nth Tree of Life and outside it are four yods on either side of the central Pillar of Equilibrium (see diagram opposite). Therefore, the number of yods below the top of the n-tree = 158n + 93 − 1 + 4 + 4 = 158n + 100.
| << Previous 1... 16 17 [18] 19 20 ...50 Next >> |