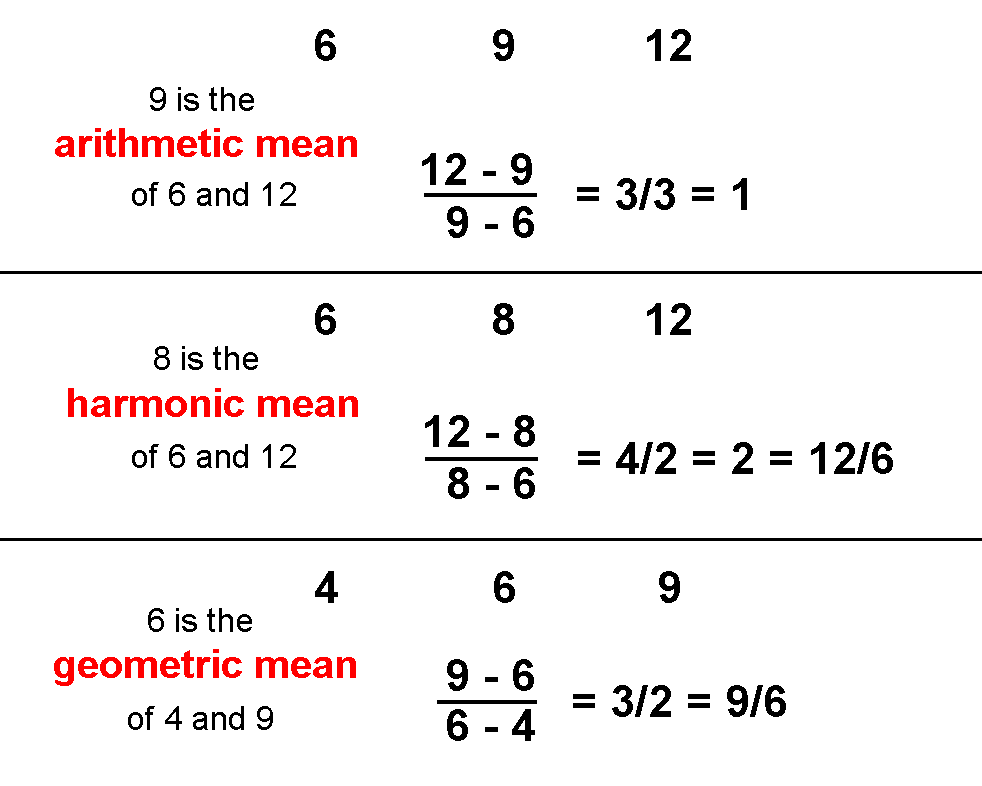
As well as an arithmetic mean A:

(b−A)/(A−a) = 1

 and a harmonic mean H:

(b−H)/(H−a) = b/a

of two numbers a & b (b>a), a geometric mean can be defined for them. Their geometric mean G is a number for which

 (b−G)/(G−a) = b/G.

For example, the geometric mean of the numbers 4 and 9 is 6 because (9−6)/(6−4) = 3/2 = 9/6. Simple algebra proves that G² = AH, so that A/G = G/H.

<< Previous    1...   9  10  [11]  12  13  ...45    Next >>

Home