4¹, 4², 4³

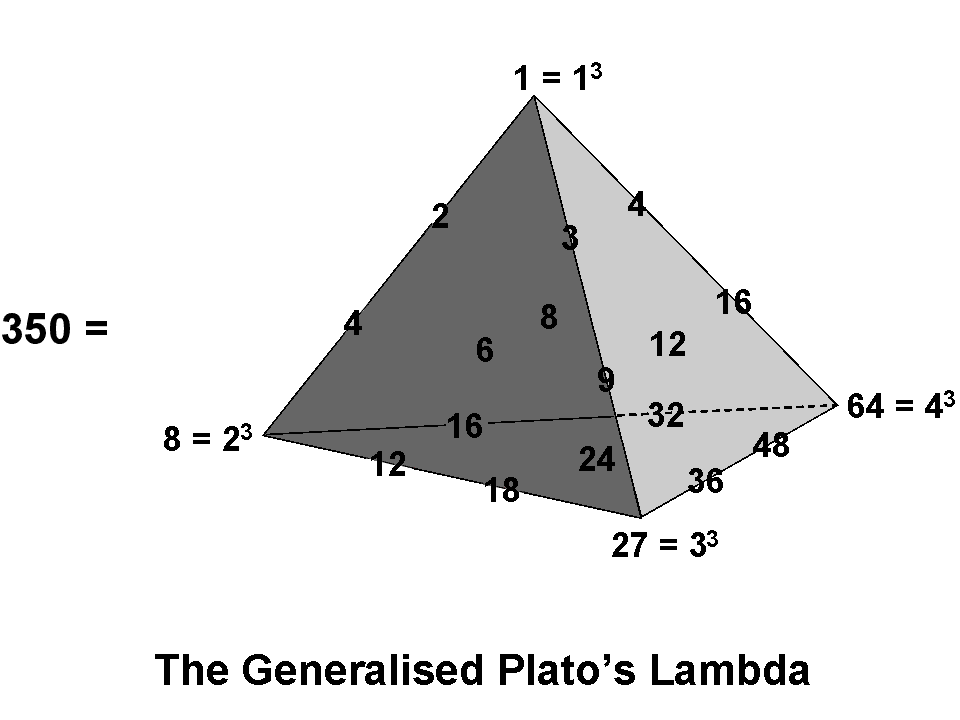
arranged along it. The resulting tetrahedron of 20 numbers, which add up to 350 and which will be called the "Tetrahedral Lambda," has the following musical virtue: the Lambda Tetractys generates the tone ratios of octaves in the Table of Nichomachus along its left-sloping side and rows parallel to it and it generates perfect fifths along its horizontal side and rows parallel to that. But, whereas the pairing of numbers separated by octaves or intervals of the perfect fifth follows the natural geometry of the array of numbers set by the extended boundary of the Lambda, the pairing of successive perfect fourths does not respect the same symmetry because it occurs in diagonal fashion across the array. The geometry of the array of integers makes the perfect fourth seem less fundamental than the perfect fifth, when really it is equally basic. Worse still, the other possible diagonal pairing of numbers whose tone ratios differ by a factor of 3 plays a relatively weak role in generating twelfths of the Pythagorean scale. The traditional construction of the tone ratios of the Pythagorean clearly lacks symmetry because the historical scheme is mathematically incomplete, being based only upon the first three of the Pythagorean set of integers 1, 2, 3 & 4. A complete scheme that included the tetrad would be one that was represented by a geometry whose symmetry did not differentiate between the status of perfect fifths and perfect fourths, which are equally fundamental divisors of the octave interval. This is what we find in the fourth face of the Tetrahedral Lambda, to be discussed next.

<< Previous    1...   5  6  [7]  8  9  ...45    Next >>

Home