| << Previous 1... 3 4 [5] 6 7 ...45 Next >> |

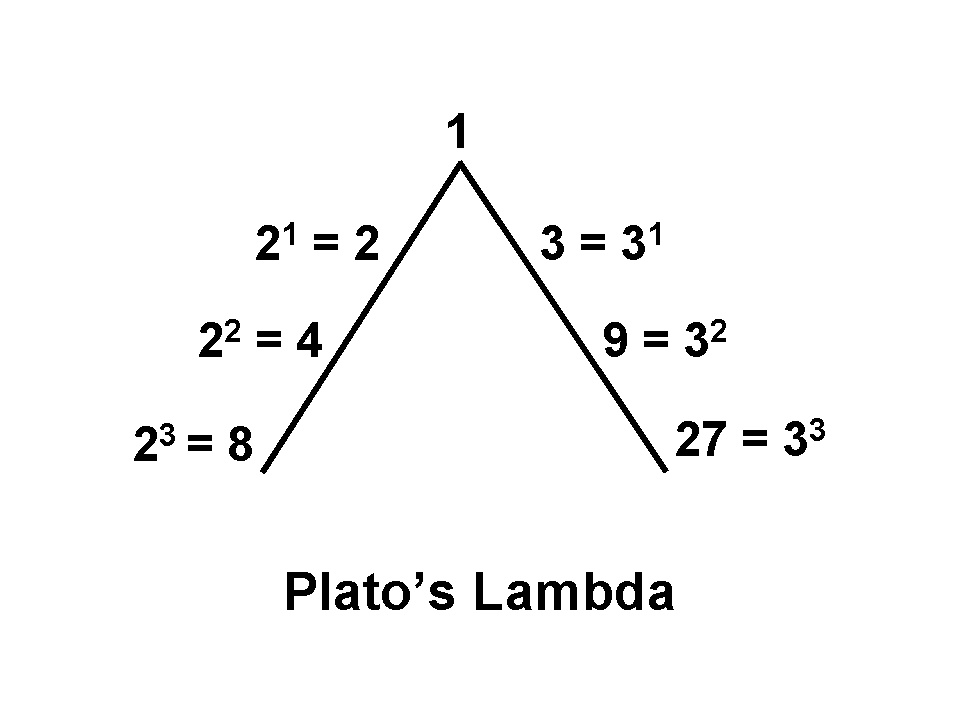
Many textbooks still state that the mathematical basis of the Western musical scale was discovered by Pythagoras. However, it is almost certain that he was taught this basis by the ancient Egyptians, with whose priests he studied for many years. In Timaeus, Plato’s treatise on Pythagorean cosmology, the central character, Timaeus of Locri (possibly a real person), describes how the Demiurge divided the World Soul into harmonic intervals. Having blended the three ingredients of the World Soul — Sameness, Difference and Existence — into a kind of malleable stuff, the Demiurge took a strip of it and divided its length into portions measured by numbers that are the first four members of two geometrical series:
1, 2, 4, 8, 16,..
and
1, 3, 9, 27, 81, ...
generated by multiplying 1 by 2 and 3. This became known as Plato’s Lambda because of its resemblance to Λ, the Greek letter lambda. Then, according to Timaeus: “he went on to fill up both the double and the triple intervals, cutting off yet more parts from the original mixture and placing them between the terms, so that within each interval there were two means, the one (harmonic) exceeding the one extreme and being exceeded by the other by the same fraction of the extremes, the other (arithmetic) exceeding the one extreme by the same number whereby it was exceeded by the other. These links gave rise to intervals of 3/2 and 4/3 and 9/8 within the original intervals. And he went on to fill up all the intervals of 4/3 (i.e., fourths) with the interval of 9/8 (the tone), leaving over in each a fraction. This remaining interval of the fraction had its terms in the numerical proportion of 256 to 243 (semitone). By this time, the mixture from which he was cutting off these portions was all used up."
The Table of Lambda, handed down to us by
Nichomachus of Gerasa (c. 60 – c. 120), a Pythagorean mathematician, makes this obscure statement
clearer. The horizontal
rows of integers in the table are
geometric progressions by 2 and the rows descending diagonally are geometric progressions by 3. Consecutive
vertical numbers are to
each other
in the ratio 3/2, defining the perfect fifth, whilst consecutive, diagonally paired numbers
(shown by arrows) are in the ratio 4/3, defining the musical fourth. The tetractys of integers at the
beginning of the Table of Lambda:
the ratio 3/2, defining the perfect fifth, whilst consecutive, diagonally paired numbers
(shown by arrows) are in the ratio 4/3, defining the musical fourth. The tetractys of integers at the
beginning of the Table of Lambda:
|
1 |
|||
| 2 | 3 | ||
|
4 |
6 |
9 |
|
| 8 | 12 | 18 | 27 |
serves as the basis of musical harmony. Using the octave of 6:12 discussed earlier, their arithmetic mean is 9, which, in relation to 6, is in the ratio 3:2. This is the perfect fifth. The harmonic mean of 6 and 12 is 8. In relation to 6, this proportion is 8:6 or 4:3, which is the perfect fourth. But 12:9 is also 4:3, that is, a perfect fourth, whilst 12:8 is also 3:2, which is a perfect fifth. The ratio 9:8 defines a whole tone interval. The central integer 6, which is absent from the series of integers:
1, 2, 3, 4, 8, 9, 27
in the Lambda, is pivotal to discussion of the mathematical proportions of the notes belonging to the musical octave for the following reason: if the integer n is chosen as the fundamental frequency, or starting note (tonic) of a musical octave, then the first overtone, or octave, has a frequency of 2n. The harmonic mean of these integers is 4n/3, which is the perfect fourth. The arithmetic mean of n and 2n is 3n/2; this is the perfect fifth. Because n has to be divisible by both 2 and 3, that is, by 6, both means are integers if n = 6N, where N = 1, 2, 3, etc. The smallest value of n defining integer values of both the perfect fourth and fifth is 6. This is why the integers 6, 8, 9 & 12 were used earlier to explain the meaning of the perfect fifth and perfect fourth.
| << Previous 1... 3 4 [5] 6 7 ...45 Next >> |