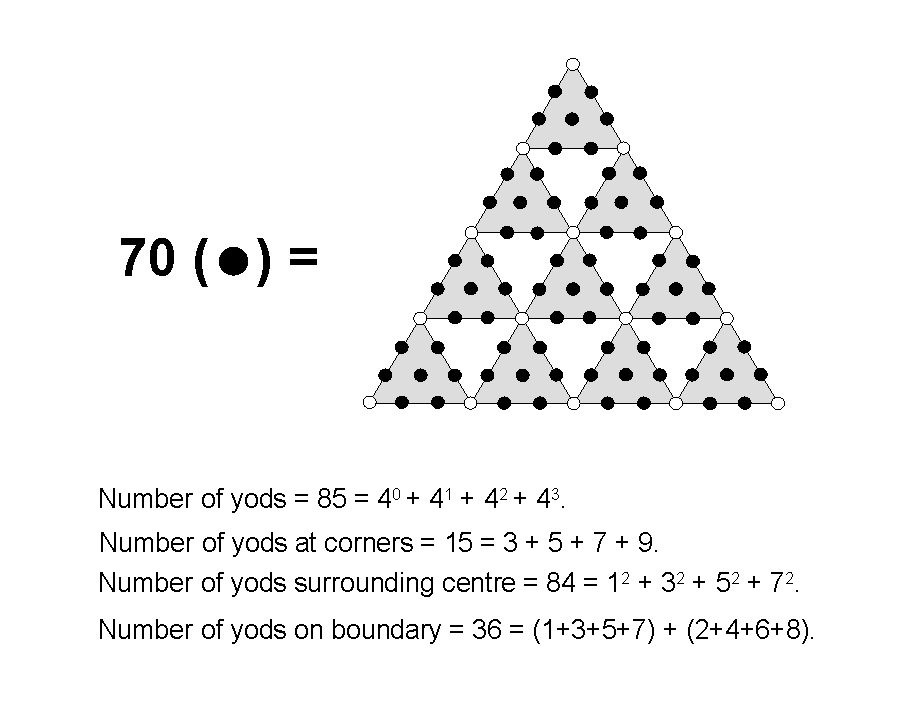
The next higher-order version of the tetractys is the 2nd-order tetractys. It has 70 hexagonal yods (shown as black dots). They correspond to the 70 yods needed to construct the Tree of Life when its 16 triangles are turned into 1st-order tetractyses (see #36). In conformity to the Tetrad Principle proposed in Article 1, the numbers of different classes of yods are the sums of the first four members of various classes of numbers.
As 4⁰ = 1², 4¹ = 2², 4² = 2⁴ and 4³ = 8², the number of yods in the 2nd-order tetractys = 85

= 4⁰ + 4¹ + 4² + 4³

= 1² + 2² + 4² + 8².

The number of corners of the 10 tetractyses = 15

= 1 + 2 + 4 + 8.

This shows how the first four terms in the geometric series 1, 2, 4, 8, .... determines properties of the 2nd-order tetractys. The number of yods surrounding its centre = 84

= 2² + 4² + 8².

The number of hexagonal yods = 70 = 84 − 14

= (2²−2) + (4²−4) + (8²−8).

This demonstrates how properties of the 2nd-order tetractys are determined by the integers 2, 4 & 8 making up the number 248, which is the number value of Raziel, the Archangel of Chokmah, and the dimension of E₈, the rank-8, exceptional Lie group at the heart of superstring theory.