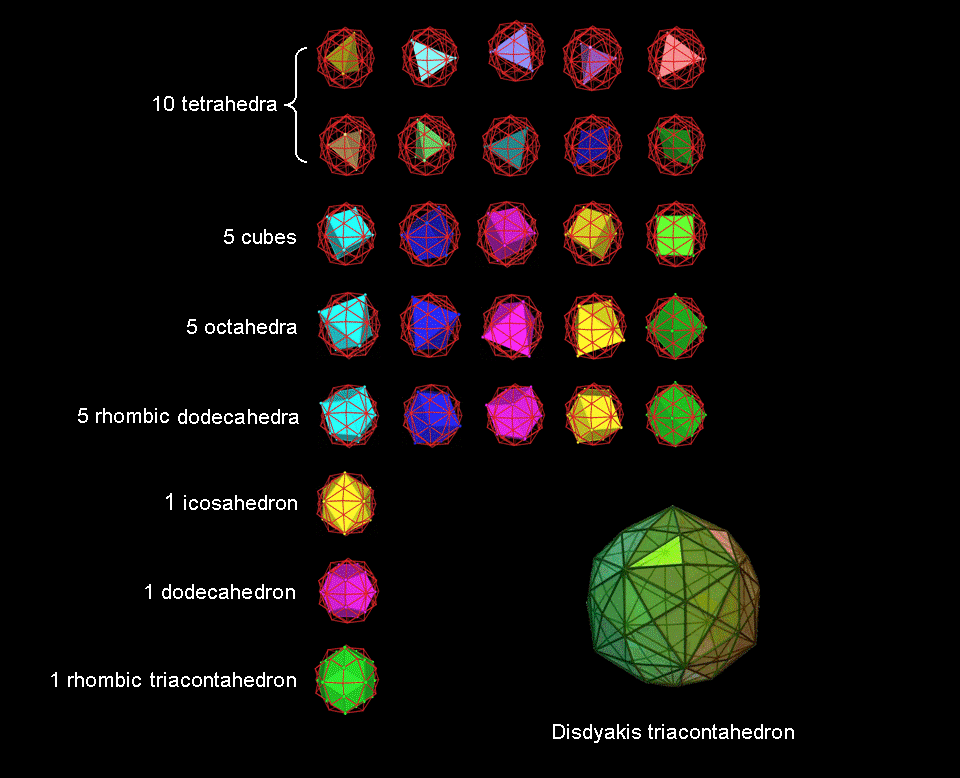
As well as the 21 Platonic solids of the first four types, the vertices of the disdyakis triacontahedron can fit five rhombic dodecahedra, a dodecahedron and one rhombic triacontahedron. In other words, 28 polyhedra of seven types can fit the disdyakis triacontahedron. The significance of this for superstrings will be discussed in Superstrings as sacred geometry/Disdyakis triacontahedron. One other polyhedron — the Archimedean solid called the icosidodecahedron — can be fitted inside the disdyakis triacontahedron. Its significance vis-à-vis the set of 28 polyhedra will be discussed here.

<< Previous    1...   7  8  [9]  10  11  ...15    Next >>

Home