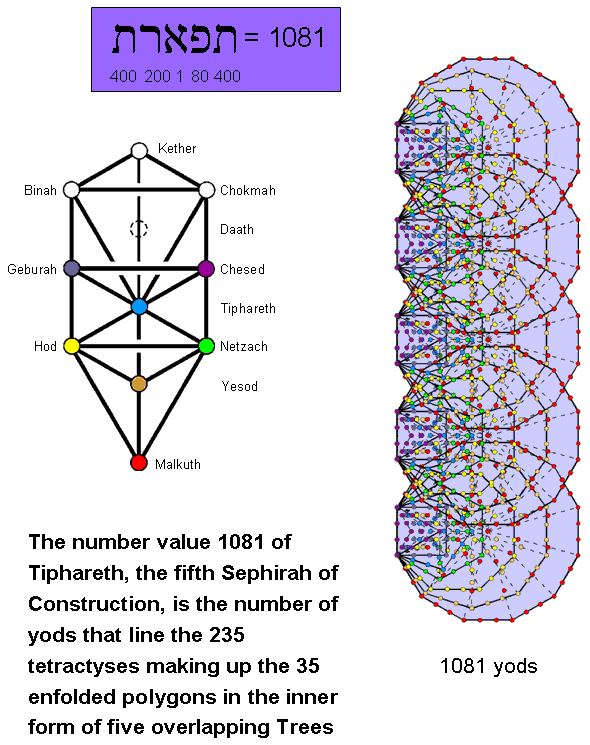
#24 The number 1081 of Tiphareth determines the inner form of five overlapping Trees of Life

The seven enfolded polygons making up each half of the inner form of the Tree of Life contain 264 yods when their 47 sectors are turned into tetractyses (see here). Therefore, as each tetractys has a hexagonal yod at its centre, (264−47=217) yods line the 88 sides of the 47 tetractyses. The topmost corner of the hexagon coincides with the lowest corner of the hexagon belonging to the set of seven enfolded polygons in the inner form of the next higher, overlapping Tree. This means that 216 boundary yods are intrinsic to the polygons enfolded in each Tree. This is the number value of Geburah (גבזרה), whose Godname ELOHA (אלה) has the number value 36 — the number of corners of the seven enfolded polygons. The number of yods lining the 47n tetractyses in the 7n polygons enfolded in the n-tree = 216n + 1, where "1" denotes the topmost corner of the hexagon that is shared with the hexagon enfolded in the next higher Tree.

Each Sephirah in the Tree of Life can be represented by a Tree, so that, for example, the lowest three Trees would represent Malkuth, Yesod & Hod, and the lowest five Trees would represent the lowest five Sephiroth up to Tiphareth. The number value of this Sephirah is 1081. According to the formula derived above, it is the number of yods lining the (47×5=235) tetractyses that make up the (7×5=35) polygons enfolded in the 5-tree: 1081 = 216×5+ 1. The meaning of the Hebrew word "Tiphareth" (also spelt "Tiferet") is "beauty." This is not merely the perfection of outer appearance but, rather, the exact balance of form and function in perfect harmony with each other.

The number of corners of the 7n polygons enfolded in the n-tree = 35n + 1. Therefore, the 35 enfolded polygons have (35×5 + 1 = 176) corners. This is a parameter of the inner Tree of Life, being the number of corners, sides & triangles making up the seven enfolded polygons, as well as the number of hexagonal yods associated with each set of seven enfolded polygons that line the sides of their 47 tetractys sectors (see here). We should expect such parameters to manifest in the properties of the inner form of five Trees of Life because, as the centre of a single Tree of Life, Tiphareth marks the half-way point in its emanation, there being five Sephiroth above it. Tiphareth is the interface, or meeting point, between the transpersonal levels of Adam Kadmon ("Atman," in Hinduism), represented by the Upper Face of the Tree of Life, and the levels of the ego or personality, which belong to the Lower Face.

There are 120 yods on the 42 sides of the seven enfolded polygons, i.e., 119 boundary yods are intrinsic to each set enfolded in successive Trees. The number of yods lining the 42n sides of the 7n polygons enfolded in the n-tree = 119n + 1. Therefore, (119×5 + 1 = 596) yods line the (42×5=210) sides of the 35 polygons enfolded in the 5-tree. As four yods lie on the root edge of each set, 20 yods make up the root edges of the five sets of polygons and (596−20=576) yods line their (210−5=205) sides outside these shared sides. 576 = 24² = 1²×2²×3²×4², demonstrating how the integers 1, 2, 3 & 4 express properties of sacred geometries. The number 576 is another parameter of the inner Tree of Life, being the number of yods that surround the centres of the two sets of seven separate polygons.*

One significance of the 5-tree for superstring physics is that, when its 67 triangles are tetractyses (67 is the number value of Binah, the third Sephirah), there are 248 yods up to Chesed of the fifth Tree (the 31st SL, where 31 is the number value of EL, the Godname of this Sephirah). 248 is the number value of Raziel, the Archangel associated with Chokmah. It is the dimension of the rank-8, exceptional Lie group E₈, one of the two symmetry groups at the heart of superstring theory. For details, see here.

Another significance of the 5-tree is that its inner form consists of (35+35=70) enfolded polygons with (840+840=1680) corners, sides & triangles that are intrinsic to it, as now explained. Each set of seven enfolded polygons consists of 47 triangles with 41 corners and 88 sides. Of these 176 geometrical elements, three corners coincide with Sephirothic corners of the outer Tree of Life and two sides of sectors of the hexagon coincide with two vertical sides of triangles in the outer Tree of Life. Hence (176−3−2−3=168) geometrical elements outside the root edge belong solely to the seven polygons of the inner Tree of Life. The (7+7) enfolded polygons comprise (168+168=336) intrinsic geometrical elements outside the root edge. The (35+35) polygons enfolded in five overlapping Trees consist of (840+840=1680) intrinsic geometrical elements. Comparing this with each whorl of the UPA/subquark superstring, which makes five revolutions around its spin axis, the five Trees signify these cycles of revolution. The 840 intrinsic geometrical elements in the 35 polygons enfolded on one side of the central axis of the outer Tree of Life correspond to the 840 circular turns in the five half-revolutions of the outer half of each helical whorl. The 840 geometrical elements in the 35 polygons enfolded on the other side of the central axis correspond to the 840 turns in the five half-revolutions of the inner half of each whorl. The five Trees representing the five revolutions of each whorl express the five Sephiroth of Construction up to Tiphareth. What is remarkable is that the gematria number value of this Sephirah is the number of yods lining the 235 tetractyses in the 35 polygons of the inner form of five Trees. Here is an amazing, undeniable connection between the paranormally determined number 1680 and the dimension 248 of E₈. It is proof of the genuine existence of micro-psi — the ability to remote-view the universe on a microscopic scale. The outer form of five overlapping Trees embodies the unified superstring force and its inner form embodies the 3-dimensional form of the superstring. The UPA/subquark superstring is an object that embodies mathematical beauty — the essence of Tiphareth.

* Proof: the number of yods in an n-gon whose n sectors are tetractyses = N(n) = 6n + 1, where "1" denotes its centre. The number of yods surrounding the centres of the seven polygons with 48 corners = ∑N(n) = 6∑n = 6×48 = 288. Therefore, (2×288=576) yods surround the centres of the two sets of seven polygons.

<< Previous    1...   22  23  [24]  25  26  ...39    Next >>

Home